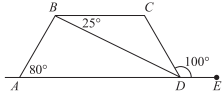
In the figure below, ABCD is a trapezoid. E lies on line AD, and angle measures are as marked. What is the measure of angle CDB?
-
Solution
To find the measure of angle CDB in the figure, it is helpful to recognize that the sides BC and AD are parallel (definition of trapezoid) and are connected by the transversal BD.
Angles CBD and ADB are alternate interior angles, and thus are equal and both measure 25°.
Because A, D, and E all lie along the same line, angle ADE = 180°.
Because angle ADE is made up of angles ADB, CDB, and CDE, the measures of these three angles add up to 180° : 25° +CDB +100° = 180°, thus the measure of angle CDB is 55°.
A square is circumscribed about a circle of a 5-foot radius, as shown below. What is the area of the square, in square feet?
-
Solution
Recall that the area of a square with side s is s2. Finding the diameter of the circle, as shown below, it is clear that the side of the square is equal to the diameter of the circle, or 2(5) = 10.
Thus the area of the square is 102, or 100 square feet
Members of the fire department lean a 26-foot ladder against a building. The side of the building is perpendicular to the level ground so that the base of the ladder is 10 feet away from the base of the building. To the nearest foot, how far up the building does the ladder reach?
-
Solution
To find out how far a 26-foot ladder reaches up a building when the base of the ladder is 10 feet away from the building, it is useful to draw a picture, as shown below:
As you can see, the ladder forms the hypotenuse of a right triangle with a length of 26, and the base of the ladder is 10 feet away from the building. Using the Pythagorean Theorem, 262 = 102 + d2, where d is the distance up the building. Simplifying, you get 676 = 100 + d2 → 576 = d2 → 24 = d.
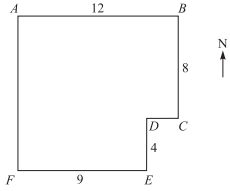
A park has the shape and dimensions, in miles, given below. The park office is located halfway between point A and point D. Which of the following is the location of the park office from point A? (Note: The park’s borders run east–west or north–south.)
-
Solution
To find the location of the park office located halfway between points A and D, it makes sense to give coordinates to the points in relation to an origin (see diagram below). In this case it makes sense to choose point F as the origin because it is in the bottom left of the figure. The first coordinate is the number of miles east of the origin, and the second coordinate is the number of miles north of the origin.
The park office is at the midpoint of the segment AD, and so the midpoint formula applies. For points with coordinates (x1, y1) and (x2, y2), the midpoint has coordinates \(\left [ \frac{(x_{1} + x_{2})}{2},\frac{(y_{1} + y_{2})}{2} \right ]\).For A (0,12) and D (9,4), the midpoint is\(\left ( \frac{[0 + 9]}{2},\frac{[12 + 4]}{2} \right )\), or (9⁄2) . However, the problem asks you to relate the location of the office to its distance and direction from point A. To do so, subtract the coordinates of point A from the coordinates of the midpoint: (9⁄2-0,8-12), or (9⁄2,-4). Thus, the location of the office relative to point A is 41⁄2 miles east and 4 miles south.
Parallelogram ABCD, with dimensions in inches, is shown in the diagram below. What is the area of the parallelogram, in square inches?Parallelogram ABCD, with dimensions in inches, is shown in the diagram below. What is the area of the parallelogram, in square inches?
-
Solution
The area for a parallelogram with base b and corresponding height h is (b)(h). For parallelogram ABCD, segment AD is the base, with length 5 + 15, or 20 inches, and the corresponding height is 12 inches. Therefore, the area is (20)(12), or 240 square inches.
The most common incorrect answer is E, which is the result of multiplying the two side lengths: (5 + 15)(13) = 20(13), or 260.