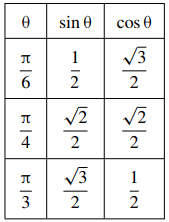
What is cos \(\frac{\pi }{12}\) given that \(\frac{\pi }{12}\) = ^π⁄₃ − ^π⁄₄ and that cos(α − β) = (cos α)(cos β) + (sin α)(sin β)?

F. ¹⁄₄
G. ¹⁄₂
H. \(\frac{\sqrt{6}+\sqrt{2}}{4}\)
J. \(\frac{\sqrt{6}+\sqrt{2}}{4}\)
K. \(\frac{\sqrt{6}+\sqrt{2}}{4}\)

Solution

If x = 3r − 4 and y = 3r + 2, which of the following expresses y in terms of x?

A. y = x + 2
B. y = x + 6
C. y = 9r + 14
D. y = 6r − 2
E. y = 3x + 14

Solution

To obtain an expression for y in terms of x when x = 3r −4 and y = 3r +2,

first solve x = 3r − 4 for r as follows:

x = 3r − 4
x + 4 = 3r
\(\frac{x + 4}{3}=r\)

Substitute that expression for r into y = 3r+2, and solve for y: y = 3\(\left [ \frac{x + 4}{3} \right ]\)+ 2, which simplifies to y = (x + 4) + 2, or y = x + 6.

A triangle, ΔABD, is reflected across the y-axis to have the image ΔA’B’D’ in the standard (x, y) coordinate plane: thus A reflects to A’ . The coordinates of point A are (m, n). What are the coordinates of point A’ ?

F. (−m, n)
G. (m, −n)
H. (−m, −n)
J. (−m, −n)
K. Cannot be determined from the given information.

Solution

To find the coordinates of vertex A after it is reflected across the y-axis,remember that a reflection across the y-axis does not change the sign of the y-coordinate but does change the sign of the x-coordinate. Therefore, you can eliminate answer choices G, H, and J. You might sketch a figure like the one below.

The reflection of A (m, n) across the x-axis is A'(−m, n). The most popular incorrect answer is J, which gives the reflection of A over the line y = x.

Which of the following is the graph, in the standard (x, y) coordinate plane, of y = \(\frac{x^{2}+3x}{x}\)?

Solution

The equation y = \(\frac{x^{2} + 3x}{x}\) can be simplified to y = \(\frac{x(x + 3)}{x}\). Therefore, the graph of this seemingly complicated equation actually looks like a line, not a parabola, so eliminate answer choices A and B. This is equivalent to y = x + 3 except when x = 0. When x = 0, the original equation is undefined. So the correct graph is y = x + 3, with a point removed where x = 0.

If f (x) = 2x² + 3, then f (x + h) = ?

F. 2x² + h²
G. 2x² + h + 3
H. 2x² + 2h² + 3
J. x² + 2xh + h² + 3
K. 2x² + 4xh + 2h² + 3

Solution

To find f(x + h) when f(x) = 2x² + 3, substitute (x + h) for x in f(x) = 2x² + 3, as follows:
f (x + h) = 2(x + h)² + 3
2(x + h)² = 2(x² + 2xh + h²) + 3
2(x² + 2xh + h²) = 2x² + 4xh + 2h² + 3

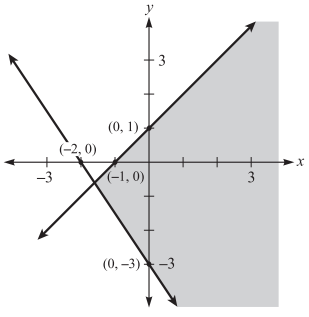
Which of the following systems of inequalities is represented by the shaded region of the graph below?

A. y ≤ x + 1 or y ≥ x − 3
B. y ≤ x + 1 and y ≥ x − 3
C. y ≤ x + 1 or y ≥ (⁻³⁄₂)x − 3
D. y ≤ x + 1 and y ≤ (⁻³⁄₂)x − 3
E. y ≤ x + 1 and y ≥ (⁻³⁄₂)x − 3

Solution

To find the system of inequalities represented by the shaded region of the graph, first find the equations of the line through (−1, 0) and (0, 1) and the line through (−2, 0) and (0, −3).

These are y = x + 1 (the y-intercept is 1) and y = (⁻³⁄₂)x − 3 (the y-intercept is −3), respectively.

Pay attention to the coordinating conjunctions, and/or.

If sin θ = ⁴⁄₅ and ^π⁄₂ < θ < π, then tan θ = ?

F. - ⁵⁄₄
G. - ⁴⁄₃
H. - ³⁄₅
J. ⁴⁄₃
K. ³⁄₄

Solution

John wants to draw a circle graph showing his friends’ favorite ice cream flavors. When he polled his friends asking each their favorite flavor of ice cream, 35% of his friends said chocolate, 20% of his friends said vanilla, 15% of his friends said strawberry, 25% of his friends said mint chocolate chip, and 5% of his friends said flavors other than those previously listed. What will be the degree measure of the vanilla sector of the circle graph?

A. 126°
B. 108°
C. 90°
D. 72°
E. 36°

Solution

You are given that 20% of John’s friends selected vanilla ice cream as their favorite flavor.

This means that 20% of the 360° in the circle will represent vanilla; 20% of 360 is equivalent to (0.20)(360°), or 72°.

If you chose one of the other answers, you may have found the degree measure of any of the other flavors.

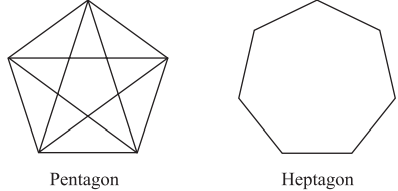
Pentagons have 5 diagonals, as illustrated below. How many diagonals does the heptagon (7 sides) below have?

F. 7
G. 12
H. 14
J. 21
K. 28

Solution

As shown below, there are 4 diagonals coming from each vertex point.

Because there are 7 vertex points, you might be tempted to conclude that there are 7×4, or 28 diagonals.

But this method counts each diagonal exactly twice.

Therefore, there are ²⁸⁄₂ , or 14 diagonals.

Which of the following is the set of all real numbers x such that x + 2 > x + 5 ?

A. The set containing only zero
B. The set containing all nonnegative real numbers
C. The set containing all negative real numbers
D. The set containing all real numbers
E. The empty set

Solution

To find the real numbers x such that x + 2 > x + 5, subtract x and 2 from both sides.

The result is 0 > 3, and because that inequality is never true, there is no solution for x.

The solution set is the empty set.

Attempted

0

Correct

0

Wrong

0

Complete