(x3 + 2x2 + 3x − 2) − (2x3 − x2 − 4) is equivalent to:
-
Solution
To solve this problem, distribute the −1 and add like terms. After distributing, the expression (x3+2x2+3x−2)−(2x3−x2−4) becomes x3+2x2+3x−2−2x3+x2+4. Combining like terms yields −x3 + 3x2 + 3x + 2.
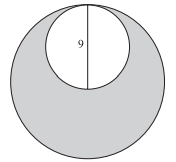
The figure below shows 2 tangent circles such that the 9- inch diameter of the smaller circle is equal to the radius of the larger circle. What is the approximate area, in square inches, of the shaded region?
-
Solution
To solve this problem, calculate the areas of the circles and subtract the area of the smaller circle from the area of the larger circle. Since the 9-inch diameter of the smaller circle is equal to the radius of the larger circle, the area of the larger circle is π(9)2 = 81π. Since the diameter of the smaller circle is 9 inches, its radius is 9⁄2 = 4.5 inches. The area of the smaller circle is then π(4.5)2 = 20.25π. The area of the shaded region is therefore (81 − 20.25)π, or 60.75π, which is approximately equal to 190.76. Remember that π is approximately equal to 3.14.
If 3 times a number x is added to 12, the result is negative. Which of the following gives the possible value(s) for x?
-
Solution
To solve this problem, write a mathematical expression for the phrase in the problem. Because when 3 times a number x is added to 12 the result is negative, 3x + 12 < 0. To solve for x, subtract 12 from both sides and divide by 3 to get x < −4.
At a summer camp, one boy and one girl will be selected to lead the weekly activities. If there are 130 boys and 145 girls at the camp, how many different 2-person combinations of 1 boy and 1 girl are possible?
-
Solution
To solve this problem, multiply the number of possibilities for each option. Since there are 130 boys and 145 girls at the camp, the number of 2-person combinations of 1 boy and 1 girl that are possible is (130)(145) = 18,850.
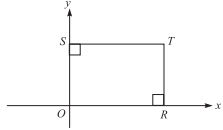
In the figure above, OS = ST and the coordinates of T are (k,5). What is the value of k?
-
Solution
Because the figure is a square (OS = ST), the x-coordinate must be the same number of units as the y-coordinate. Also, since T is in Quadrant I, the x-coordinate must be positive. Eliminate answer choices A, B, and C. Since the y-coordinate is 5, the x-coordinate, k, must also be 5.
The average (arithmetic mean) of a and b is 6 and the average of a, b, and c is 11. What is the value of c?
-
Solution
To solve this problem, first recognize that \(\frac{a + b}{2}\)= 6, which means that a + b = 12. Also, \(\frac{a + b + c}{3}\) = 11, which means that a + b + c = 33. Therefore, 12 + c = 33, so c must equal 33 − 12, or 21.
A house painter charges $24.00 per hour for a painting job that requires more than 5 hours to complete. For any job requiring 5 hours or less, the house painter charges a flat fee of $100. If n represents the number of hours the job requires, which of the following expressions gives the charge, in dollars, for a job requiring more than 5 hours to complete?
-
Solution
Since the house painter charges $24.00 per hour for a painting job that requires more than 5 hours to complete and n represents the number of hours the job requires, the charge, in dollars, for a job requiring more than 5 hours to complete can be expressed as (24.00)(n), or 24n. This problem tested your ability to disregard irrelevant information.
A system of linear equations is shown below.
4y − 2x = 8
4y + 2x = 8
Which of the following describes the graph of this system of linear equations in the standard (x, y) coordinate plane?
-
Solution
To solve this problem, it would be helpful to make the properties of both lines more evident by converting them to slope-intercept form (y = mx + b, where m is the slope and b is the y-intercept). To start, adding 2x to both sides and dividing both sides of the equation 4y − 2x = 8 by 4 yields y = x⁄2 + 2. Similarly, subtracting 2x from both sides and dividing both sides of the equation 4y + 2x = 8 by 4 yields y = −x⁄2 + 2. The relationship between y = x⁄2 + 2 and y = −x 2 + 2 is that they are lines that share the same y-intercept, yet they have opposite slopes (1⁄2 and −1⁄2). Therefore these are two distinct intersecting lines.
If 42⁄5 = a − 12⁄3, then a = ?
-
Solution

What is the least common multiple of 40, 70, and 60?
-
Solution
To solve this problem, start by finding the least common multiple of 60 and 70, which is 420. However, 420 is not a multiple of 40. Next try 2 × 420, which is 840. The number 840 is still a multiple of 60 and 70 and is also a multiple of 40. Another possible strategy for this problem would be to start with the smallest answer choice (you are asked for the least common multiple) and stop when you find an answer choice that is evenly divisible by 40, 60, and 70.
