ACT
Practice Test 47
Previous Solution Next
\(\frac{2r}{3}+\frac{4s}{5}\) is equivalent to:

  • Solution

A line in the standard (x, y) coordinate plane is parallel to the x-axis and 5 units below it. Which of the following is an equation of this line?

  • Solution

    A line parallel to the x-axis is horizontal. Horizontal lines have the equation y = b, where b is a constant, and represents the y-intercept. Therefore, a line in the standard (x, y) coordinate plane is parallel to the x-axis and 5 units below it will have the equation y = −5.

Which of the following is a factor of the polynomial x1⁄2 + 3x − 18?

  • Solution

    To solve this problem, factor the polynomial x2+3x−18. To do so, think of x2+3x−18 as (x + ?)(x − ?). To fill in the question marks, find two numbers that multiply to equal −18 and add up to 3. One such pair of numbers is 6 and −3. To check, make sure that (x + 6)(x − 3) = x2 +3x −18, which it does. Of the answer choices, only (x + 6) is a factor.

If 3(x − 2) = −7, then x =?

  • Solution

    To solve for x, distribute the 3 in the equation 3(x−2) = −7 to get 3x−6 = −7.

    Adding 6 to both sides of the equation yields 3x = −1, or x = −1⁄3.

In ABC below, AB ≅ BC, and the measure of ∠B is 55°. What is the measure of ∠C?

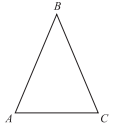
  • Solution

    To solve this problem, use the fact that since AB ≅ BC, triangle ABC is isosceles.

    In isosceles triangles, the angles opposite equal sides have equal measure.

    In this triangle, since angles A and C are opposite sides AB and BC, they have equal measure.

    Because the sum of the angles in a triangle is always 180°, the sum of the measures of angles A and C is 180° less the measure of angle B, or 180◦ −55◦ = 125°.

    Therefore, since angles A and C have equal measure, they both equal \(\frac{125^{\circ}}{2}\) = 62.5°.

On the real number line below, numbers decrease in value from right to left, and Y is positive. The value of X must be:

  • Solution

    It is given that on the number line, numbers decrease in value from right to left, meaning that numbers on the right are greater than numbers on the left.

    Since no indication of the location of 0 is given in the picture, no assumption about negative or positive can be made.

    Simply because numbers on the right are greater than numbers on the left and Y is to the right of X, Y must be greater than X.

    Put another way, X must be less than Y, answer choice D.

For what value of a is the equation 3(a + 5) − a = 23 true?

  • Solution

    To find the value of a such that the equation 3(a + 5) − a = 23 is true, solve 3(a + 5) − a = 23 for a:
    3a + 15 − a = 23
    2a + 15 = 23
    2a = 8
    a = 4

The balance of Juan’s savings account quadrupled during the year. At the end of the year, Juan withdrew $300, and the resulting balance was $400. What was the balance in the account before it quadrupled?

  • Solution

    When Juan withdrew $300, the resulting balance was $400, making the balance of the account before withdrawal $400 + $300, or $700.

    Since the balance of Juan’s savings account quadrupled during the year, the balance, b, in the account before it quadrupled is represented by 4b = 700.

    Therefore b = \(\frac{700}{4}\) , or $175.

Assume that the statements in the box below are true.

Considering only the statements in the box, which of the following statements must be true?

Which point in the standard (x,y) coordinate plane below has the coordinates (2,−5)?

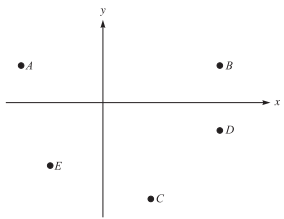
  • Solution

    Since the point (2,−5) has a positive x-coordinate, it will have a placement to the right of the y-axis.

    Similarly, since the point has a negative y-coordinate, it will have a placement below the x-axis.

    Of the possible points, only C and D fit such a description.

    Of the two points, C appears to have a y-coordinate with greater magnitude than its x-coordinate, which would correspond to the point (2,−5).

Attempted

0

Correct

0

Wrong

0

Complete
Math Solved Practice Test
Attempted
Wrong
Correct