A formula for calculating simple interest is I = Pr, were I is the interest earned in dollars, P is the principal or original investment, and r is the fixed rate of interest. If the amount of interest earned is $2.25 and the interest rate is 3%, what is P?
-
Solution
You are given two of the three unknowns; plug I = 2.25 and r = 0.03 (the decimal equivalent of 3%) into the formula I = Pr:
2.25 = P(0.03)
Solve for P:
P = 75
What is the slope of the line pictured in the standard (x,y) coordinate plane below that passes through (1,3) and (5,5) in the standard (x,y) coordinate plane?
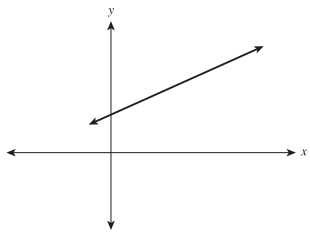
-
Solution
By definition, the slope of any line is \(\frac{change\: in\: y}{change\: in\: x}\).
The question asks you to determine the slope from two points on the graph, so divide the change in y between the two points given by the change in x between the two points given:
\(\frac{y_{1} − y_{2}}{x_{1} − x_{2}}=\frac{5 − 3}{5 − 1}=\frac{2}{4}\)
2⁄4 = 1⁄2
The figure below represents a solution set for which of the following inequalities?
![]()
-
Solution

In the standard (x,y) coordinate plane, what is the x intercept of a line that has a slope of 2⁄3 and passes through the point (−2,2)?
-
Solution
By definition, the slope of any line is \(\frac{change\: in\: y}{change\: in\: x}\).
The question gives the slope of the line as 2⁄3, which means that for every positive change of 2 in y, there is a positive change in x of 3.
The question asks you to determine the x-intercept, or where y = 0. You are given a point on the line, (−2, 2).
Use the point-slope form of a line, y = mx + b, set y to 0, m to 2⁄3, and solve for x:
(0 − 2) = 2⁄3(x + 2)
−2 = 2⁄3x + 4⁄3
−6⁄3 = 2⁄3x + 4⁄3
−\(\frac{10}{3}\) = 2⁄3x, x = −5. The x-intercept is (−5,0).
In the figure below, both circles are centered around X. The length of XY is 2 units and the length of XZ is 6 units. If the smaller circle is cut out of the larger circle, how much of the area, in square units, of the larger circle will remain?
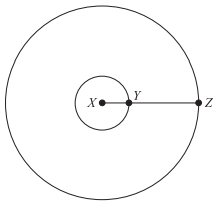
-
Solution
Since you are given the radii of both circles, you can find the area of both circles.
The radius of the smaller circle is 2, so the area is π(2)2, or 4π.
The radius of the larger circle is 6, so the area is π(6)2 = 36π.
Since the smaller circle is removed from the larger circle, simply subtract the area of the smaller circle from the area of the larger circle: 36π − 4π = 32π.
Fifty (50) households were surveyed to determine the number of TVs in each of the households. The number of TVs in each household is shown in the chart below. What is the average number of TVs per household for these 50 households?

-
Solution
This question is asking you to determine a weighted average. To answer the question, first calculate the total number of TVs:
(2 × 5), (3 × 20), (4 × 15), and (5 × 10).
Next, add these values together and divide by the total number of houses (50):
\(\frac{(2 × 5) + (3 × 20) + (4 × 15) + (5 × 10)}{50}\)
\(\frac{10 + 60 + 60 + 50}{50}=\frac{180}{50}=3.6\)
What is the slope of the line determined by the equation 2x − 3y = 6?
-
Solution
Find the slope by putting 2x − 3y = 6 into the slope-intercept form (y = mx + b where m is the slope):
−3y = −2x + 6
y = 2⁄3x − 2. The slope is 2⁄3.
In the standard (x,y) coordinate plane, what is the center of a circle with the equation (x − 2)2 + (y + 1)2 = 4?
-
Solution
The formula for a circle is (x − a)2 + (y − b)2 = r2, where (a, b) is the center of the circle and r is the radius of the circle.
The question gives you the equation of the circle as (x − 2)2 + (y + 1)2 = 4.
So, a is 2 and b is −1.
The center of the circle, (a, b), is (2, −1).
In an isosceles right triangle, the hypotenuse is 12. What is the length of one (1) of the sides?
-
Solution
By definition, an isosceles triangle has a hypotenuse, c, and two legs, a and b,that are congruent.
Use the measure of the hypotenuse given in the problem (12), and the Pythagorean theorem, a2 + b2 = c2, to determine the length of one of the legs.
Remember that a = b:
a2 + a2 = 122
2a2 = 144
a2 = 72
a = \(\sqrt{72}\)
The \(\sqrt{72}\) can be reduced to 6√2.
If x2 −3 ≤ 13, what is the greatest real value that x can have?
-
Solution
Here, you must first solve the inequality:
x2 − 3 ≤ 13
x2 ≤ 16
Because a negative number squared will yield a positive result, you must consider both the positive and negative values of x.
x ≤ 4 and x ≥ −4. The greatest real value of x is if x = 4.
