A total of f men went on a fishing trip. Each of the r boats that were used to carry the fishermen could accommodate a maximum number of m passengers. If one boat had 5 open spots and the remaining boats were filled to capacity, which of the following expresses the relationship among f , r, and m?

F. rm + 5 = f
G. rm − 5 = f
H. r + m + 5 = f
J. rf = m + 5
K. rf = m − 5

Solution

If each of the r boats that were used to carry the fishermen could accommodate a maximum number of m passengers, the total capacity of fisherman is rm.

Since only one boat had 5 open spots and the remaining boats were filled to capacity, the number of fishermen was 5 less than the total capacity, or rm − 5. Thus f = rm − 5.

If cos x = ⁵⁄₇ and tan x = ⁴⁄₅, what is sin x?

A. ⁴⁄₇
B. ⁷⁄₉
C. ⁵⁄₄
D. ⁹⁄₇
E. ⁷⁄₅

Solution

The cosine of any acute angle is the length of the adjacent side divided by the hypotenuse \(\left ( \frac{adj}{hyp} \right )\).

The tangent of any acute angle is the length of the opposite side divided by the length of the adjacent side \(\left ( \frac{opp}{adj} \right )\).

The sine of any acute angle is the length of the opposite side divided by the hypotenuse.

You are given that cos x = ⁵⁄₇\(\left (\frac{adj}{hyp} \right )\) and tan x = ⁴⁄₅\(\left (\frac{opp}{adj} \right )\).

Sin x must equal ⁴⁄₇ \(\left (\frac{opp}{hyp} \right )\).

Given the graph below in the standard (x,y) coordinate plane, the slope of line a is ma and the slope of line b is mb. Which of the following statements about the slope of lines a and b is true?

F. m_a = \(\frac{-1}{m_{b}}\)
G. m_a + m_b = 0
H. −¹⁄₂m_a = m_b
J. m_a − 1 = m_b
K. m_a − m_b = 0

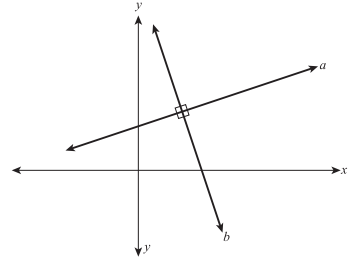
Solution

Based on the figure, line a and line b are perpendicular lines, because they intersect to form right angles.

Perpendicular lines have slopes that are negative reciprocals. In other words, if the slope of line a is m_a, the slope of line b is ⁻¹⁄_{m_a}.

You are given that the slope of line b is m_b,so m_b = ⁻¹⁄_{m_a}.

This is equivalent to ma = ⁻¹⁄_{m_b}.

In the figure below, P and Q lie on the circle R, which has a radius of 9. If the angle PRQ is 120◦, what is the area of sector PRQ?

A. 3π
B. 9π
C. 27π
D. 81π
E. 243π

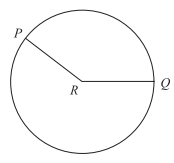
Solution

There are 360◦ in a circle.

∠PRQ is 120◦, so the sector makes up 120◦÷360◦, or ¹⁄₃, of the area of the circle.

To answer this question, determine the area of the entire circle and take ¹⁄₃ of the result to find the area of the sector.

The formula for the area of a circle is A = πr².

The radius, 9, is given in the problem, so plug 9 into the formula:

= π(9)²

= 81π

You know that the sector is ¹⁄₃ of the circle, so divide the area of the entire circle by 3:

81π ÷ 3 = 27π

Which of the following intervals contains the solution to the equation x − 2 =\(\frac{2x + 5}{3}\)?

F. −6 < x < 11
G. 11 ≤ x < 15
H. 6 < x ≤ 10
J. −5 < x ≤ −3
K. −11 ≤ x ≤ −2

Solution

First, solve the equation for x:

x − 2 = \(\frac{2x + 5}{3}\)

3x − 6 = 2x + 5

x = 11

Only the interval 11 ≤ x<15 contains 11, so answer choice G must be correct.

In the figure below, the lengths of the sides of triangle BAC are as shown. \(\overline{BD}\) bisects side \(\overline{AC}\). What is the length of DC?

A. √3
B. 2
C. 3
D. 2√5
E. 4

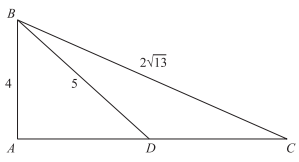
Solution

The first step in answering this question is to use the Pythagorean Theorem,a² + b² = c², to determine the length of AB.

BD, the line that bisects AC, creates a second triangle, BAD. Use the length of lines BD and AD to determine the length of AD (let AD = a).

a² + 4² = 5²

a² + 16 = 25

a² = 9

a = 3

Because you known that BD bisects AC, you know that the length of DC must also equal 3.

What values of x make the inequality −5x − 7 > 3x + 1 true?

F. x > 1
G. x < 8
H. x < −1
J. x > −4
K. x < 2

Solution

The first step in answering this question is to rearrange the terms so that the x’s are all on one side of the inequality,

−5x − 7 > 3x + 1:

−7 > 8x + 1

The next step is to get x alone:

−8 > 8x

−1 > x, which is equivalent to x < −1.

José is building a scale model of a sailboat, complete with a main sail. The actual sailboat’s main sail measures 56 feet high with a base of 32 feet. If the model sailboat’s main sail has a base of 8 inches, how tall will the model’s main sail be, in inches?

A. 14
B. 28
C. 32
D. 56
E. 112

Solution

A line in the standard (x,y) coordinate plane has a slope of ²⁄₃ and passes through points (3,4) and (t,−2). What is the value of t?

F. 3
G. 2
H. 0
J. −2
K. −6

Solution

In the figure below, PQRS is a rectangle with sides of lengths shown. X is the midpoint of \(\overline{SR}\). What is the perimeter of triangle PXQ?

A. 10
B. 4√2 + 12
C. 3√2 + 12
D. 8√2 + 8
E. 4√2 + 4

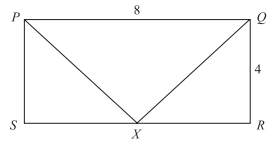
Solution

You know that QRX is a right triangle, and QR = 4.

Since X is the midpoint of SR, which is 8, RX must equal 4.

QX = 4√2, since RX is an isosceles triangle where the hypotenuse is √2 times the measure of the legs of the triangle.

Since QX = 4√2, PX must also equal 4√2.

So, the perimeter of triangle PXQ is 8 + 4√2 + 4√2, or 8√2 + 8.

Attempted

0

Correct

0

Wrong

0

Complete