ACT
Practice Test 13
Previous Solution Next

Points B and C lie on segment AD as shown below. The length of segment AD is 25 units; the segment AC is 19 units long; and the segment BD is 14 units long. How many units long, if it can be determined, is the segment BC?

  • Solution

    You are given that the length of AC is 19 units and the length of BD is 14 units. In addition, points are along segment AD as shown in the problem. Segment BC is the intersection of segment AC and segment BD. Therefore, the sum of the lengths AC and BD is the same as the sum of the lengths AD and BC. Substitute the actual lengths in AC + BD = AD + BC as follows: 19 + 14 = 25 + BC → 33 = 25 + BC → 8 = BC.

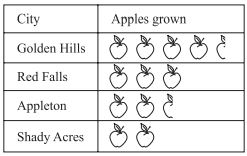
The table below shows the number of pounds of apples grown last year in 4 cities. (Each whole apple on the graph represents 1,000 pounds of apples.) According to the graph, what fraction of the apples grown in all 4 cities were grown in Appleton?

  • Solution

    To find the fraction of apples grown in Appleton, divide the number of apples grown in Appleton by the total number of apples grown. The table below shows the conversion of apple symbols to numbers for the 4 cities, as well as the total number of apples grown.

    The fraction of apples grown in Appleton is \(\frac{2,500}{12,000}\), or 5⁄24.
    If you selected answer choice D, the most common incorrect answer, you probably used the number grown in Appleton divided by the total number of apples from the other 3 towns only.

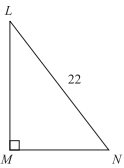
The hypotenuse of the right triangle LMN shown below is 22 feet long. The cosine of angle L is 2⁄3. How many feet long is the segment LM?

  • Solution

    To find the length of the segment LM in ΔLMN, where the length of the hypotenuse is 22 and the cosine of angle L is 3⁄4, use the definition of cosine, which is the ratio of the length of the adjacent side to the length of the hypotenuse. In LMN, the cosine of angle L is the ratio of the length of segment LM to the length of the hypotenuse. Substitute the length of the hypotenuse and solve for LM, as follows:

    3⁄4 = \(\frac{LM}{20}\)

    4 × LM = 22 × 3

    LM = \(\frac{66}{4}\), or 16.5, answer choice G.

After a snowstorm, city workers removed an estimated 12,000 cubic meters of snow from the downtown area. If this snow were spread in an even layer over an empty lot with dimensions 62 meters by 85 meters, about how many meters deep would the layer of snow be?

  • Solution

    To find the uniform depth, use the formula for volume, V, of a rectangular prism with the height h, length l, and width w, V = (l)(w)(h).

    Substitute the given values for the variables and solve for

    h: 12,000 = (62)(85)(h), or 12,000 = 5, 270h.

    Thus h = \(\frac{12,000}{5,270}\) , or about 2.277, which is between 2 and 3.

The length L, in meters, of a spring is given by the equation L = (2⁄3)F+0.05, where F is the applied force in newtons. Approximately what force, in newtons, must be applied for the spring’s length to be 0.23 meters?

  • Solution

    To find the force F (in newtons) corresponding to the spring length, L, of 0.23 meters when the relationship is given by the equation L = (2⁄3)F + 0.05,

    first substitute 0.23 for L to get 0.23 = (2⁄3)F + 0.05.

    Next, subtract 0.05 from both sides to get 0.18 =(2⁄3)F.

    Finally, multiply by 2⁄3), since dividing by a fraction is equal to multiplying by its reciprocal, to arrive at 0.27 = F

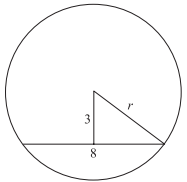
A chord 8 inches long is 3 inches from the center of a circle, as shown below. What is the radius of the circle, to the nearest tenth of an inch?

  • Solution

    To find the radius, use the right triangle shown in the diagram. Half of the length of the chord is 4 inches, which is the length of one leg.

    The other leg is 3 inches long, and the hypotenuse is r inches long.

    (Note: this is a right triangle because the distance between a point and a line is measured perpendicular to the line.) Use the Pythagorean Theorem, as follows: r2 = 32 + 42 → r2 = 9 + 16 → r2 = 25 → r = 5 inches.
    If you selected answer choice E, you probably used 8 and 3 for the leg lengths and got r2 = 73,which makes r equivalent to about 8.5 inches.

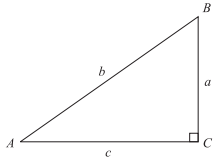
For the right triangle ABC shown below, what is tan B?

  • Solution

    To find tan B in ΔABC, take the ratio of the length of the opposite side to the length of the adjacent side: AC to BC = c to a, or c⁄a.
    Answer choice F is cos B; answer choice G is cot B; answer choice H is sec B; answer choice K is sin B.

Which of the following is a solution to the equation x2 + 25x = 0?

  • Solution

    To solve the quadratic equation x2 + 25x = 0 for x, factor out an x on the left side of the equation: x(x + 25).

    Now, apply the zero product rule: x = 0 or x + 25 = 0.

    If x + 25 = 0, then x = −25, which is answer choice E.

     

What is the slope-intercept form of 6x − 2y − 4 = 0?

  • Solution

    The slope-intercept form of the equation of a line states that y = mx + b. To find the slope-intercept form of the equation 6x − 2y − 4 = 0, you must isolate y on the left side of the equation, as follows:
    6x − 2y − 4 = 0
    −2y − 4 = −6x
    −2y = −6x + 4
    y = 3x − 2
    If you selected answer choice J, you probably forgot to switch the signs when dividing by −2.
    It is crucial to multiply all terms on both sides ofthe equation to arrive at a correct answer.

For all positive integers a, b, and c, which of the following expressions is equivalent to a⁄c?

  • Solution

    To find an equivalent expression for a⁄c, either multiply or divide both the numerator and denominator by the same value.

    Because the question asks for all positive integers a, b, and c, and you are looking for an expression that is equivalent to a⁄c, multiply a⁄c by b⁄b to get \(\frac{(a \times b)}{(c \times b)}\), answer choice A

Attempted

0

Correct

0

Wrong

0

Complete
Math Solved Practice Test
Attempted
Wrong
Correct