A triangle, ΔABD, is reflected across the line y = x to have the image ΔA’B’D’ in the standard (x, y) coordinate plane: thus A reflects to A’. The coordinates of point A are (m, n). What are the coordinates of point A’?
-
Solution
The image of a point (x, y) reflected across the line y = x will have coordinates (y, x). If the coordinates of point A are (m, n), then the coordinates of point A'are (n, m).
In the right triangle below, YZ = 10 units, and XZ = 4 units. What is sin Z?

-
Solution

A computer repair person charges $50.00 per hour, plus an additional mileage fee. The charge for mileage varies directly with the square root of the number of miles traveled. If one hour plus 25 miles traveled costs $140.00, what is the total amount charged for one hour plus 36 miles traveled?
-
Solution
You are given that a computer repair person charges $50.00 per hour, plus an additional mileage fee which varies directly with the square root of the number of miles traveled. Therefore, the total fee can be expressed as 50h + k√m, where h is the number of hours worked, m is the number of miles traveled, and k is some constant. Since one hour plus 25 miles traveled costs $140, 140 = 50 + k\(\sqrt{25}\) = 50 + 5k. Since 140 = 50 + 5k, k = 18. The total amount charged for one hour plus 36 miles traveled is 50+18\(\sqrt{36}\) = 50 + 18(6) = 50 + 108 = $158.00.
For some real number n, the graph of the line y = (n + 1)x + 6 in the standard (x, y) coordinate plane passes through (4,8). What is the value of n?
-
Solution
Given that the graph of the line y = (n + 1)x + 6 in the standard (x,y) coordinate plane passes through (4,8), plug in the values of the point (4,8) into the equation and solve for n. Substituting (4,8) into y = (n+1)x+6 yields 8 = (n+1)(4)+6 = 4n+10. To solve 8 = 4n+10, subtract 10 from both sides and divide by 4 to get n = −1⁄2.
Which of the following expresses the number of miles a runner must travel in a 4-lap race where the course is a circle of radius m miles?
-
Solution
The number of miles a runner must travel in a 4-lap race where the course is a circle of radius m miles will be equal to 4 times the circumference of the circle. Since circumference is 2πr, where r is the radius, the circumference is 2πm. Since it is a 4-lap race, the total number of miles traveled is 4(2πm), or 8πm.
When graphed in the standard (x, y) coordinate plane,the lines x = −5 and y = x − 5 intersect at what point?
-
Solution
To solve this problem, substitute the equation x = −5 into y = x − 5 to find the y-coordinate at which the lines x = −5 and y = x −5 intersect (the x-coordinate is −5 because it is given that x = −5). Thus y = (−5)−5 = −10. The point of intersection is (−5,−10).
A horse eats 12 bales of hay in 5 days. At this rate, how many bales of hay does the horse eat in 5 + x days?
-
Solution
If a horse eats 12 bales of hay in 5 days, the average rate is \(\frac{12}{5}\) bales per day. At this rate, the number of bales of hay that the horse eats in 5 + x days is the 12 bales for the 5 days plus \(\frac{12}{5}\)
bales per day after that, or (\(\frac{12}{5}\))x.
Thus the total is 12 + (\(\frac{12}{5}\))x, or 12 + \(\frac{(12x)}{5}\).
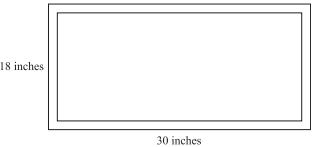
The picture shown below has a uniform frame-width of 5⁄8 inches. What is the approximate area, in square inches, of the view able portion of the picture?
-
Solution
Since the width of the frame is 5⁄8 or 0.625 inches, the length of the view able portion is 30 − 2(0.625) = 28.75 inches and the width is 18 − 2(0.625) = 16.75 inches. Thus, the area is 28.75 × 16.75 = 481.56 square inches
If 38x = 813x−2, what is the value of x?
-
Solution
To solve this problem, recall that 81 = 34. Then 813x−2 = (34) 3x−2 = 34(3x−2). Further, if 38x = 813x−2, then 38x = 34(3x−2) and 8x = 4(3x − 2), 12x − 8. Subtracting 8x from both sides and adding 8 to both sides yields 4x = 8, or x = 2.
What is the slope of a line that is parallel to the line determined by the equation 5x − 4y = 8?
-
Solution
Parallel lines have equal slopes. To find the slope of a line that is parallel to the line determined by the equation 5x−4y = 8, put the equation in slope-intercept form (y = mx + b). To do so, first subtract 5x from both sides to get −4y = −5x + 8. Then divide by −4 to get y = \(\frac{5x}{4}\)-2.thus the slope is 5⁄4.
