ACT
Practice Test 66
Previous Solution Next

  \(\frac{\left ( \frac{5}{4} \right )\left ( \frac{4}{3} \right )}{\left ( \frac{1}{3} \right )\left ( \frac{2}{3} \right )}\)=?

  • Solution

    When multiplying fractions, multiply the numerators together and then multiply the denominators together and then reduce.

    (5⁄4)(4⁄3) = \(\frac{20}{12}\) = 5⁄3

    (1⁄3)(2⁄3) =2⁄9

    When dividing fractions, multiply the first fraction by the reciprocal of the second fraction and reduce.

    (5⁄3) ÷ (2⁄9)

    (5⁄3)(9⁄2)

    \(\frac{45}{6}=\frac{15}{2}\)

Given the system of equations below, x = ?
y + 3x = 9
2x − 1⁄3y = 6

  • Solution

    The first step in answering this question is to rearrange one of the given equations so that it is expressed in terms of y:

    y + 3x = 9

    y = −3x + 9

    To solve for x, the next step is to plug −3x + 9 in for y in the second equation:

    2x − 1⁄3(9 − 3x) = 6

    2x − 3 + x = 6

    3x = 6 + 3

    x = 3.

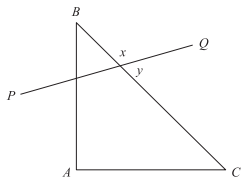
In the figure below, triangle ABC is a 30–60–90 right triangle. If angle x measures 125°, what is the measure of angle y?

  • Solution

    Angles x and y are supplementary angles, so their sum is 180°.

    Angle x is given as 125°, so angle y is 180° − 125° = 55°.

There are 32 ounces in a quart. If 2 quarts of milk costs $2.65, what is the cost of milk per ounce, to the nearest cent?

  • Solution

    Given 32 ounces in a quart, you can calculate that there are 64 ounces in 2 quarts.

    2 quarts, or 64 ounces, costs $2.65.

    Divide $2.65 by 64 (2.65 ÷ 64) to get $0.04 per ounce.

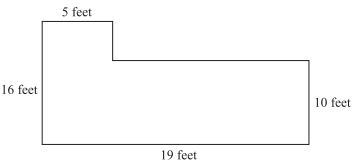
A floor has the dimensions shown below. How many square feet of tile are needed to cover the entire floor?

  • Solution

    First, divide the region into two rectangles, as shown:

    The first rectangle is 5 feet by 16 feet. The second rectangle is 10 feet by 14 feet (19 feet minus 5 feet).

    The area of a rectangle is calculated by multiplying the length by the width.

    Add the areas together:

    Rectangle 1: (5)(16) + Rectangle 2: (10 (14) = 220 square feet.

Jordan went for a 3.5-mile jog on Monday that took him 40 minutes. If on Tuesday Jordan jogs at the same rate of speed, how far will he jog in 60 minutes?

  • Solution

    To solve this problem, set up a proportion: 3.5 miles is to x miles as 40 minutes is to 60 minutes.

    Cross-multiply and solve for x:

    \(\frac{3.5}{x}=\frac{40}{60}\)

    40x = 210

    x = 5.25

A rectangular parking lot has an area 315 square yards.Its length (l) is 3 times its width (w). Which of the following equations could you use to determine the width of the parking lot?

  • Solution

    The formula for the area of a rectangle is A = l × w, with l as the length and w as the width.

    The question states that the length of the parking lot is three times as long as the width.

    If w is the width, then the length is 3 × w, or 3w.

    Therefore, the area of the parking lot is 3w × w = 315.

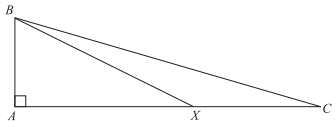
In the figure below, triangles ABC and ABX are both right triangles. If the length of \(\overline{AB}\) is 6 units, the length of \(\overline{BX}\) is 10 units, and the length of \(\overline{XC}\) is 4 units, what is the length of \(\overline{BC}\)?

  • Solution

    You are given that AB = 6 and BX = 10. Use the Pythagorean Theorem to solve for AX:

    62 + (AX)2 = 102

    (AX)2 = 64

    AX = 8

    If AX = 8 and XC = 4, then AC must equal 12.

    Given that AB = 6 and AC = 12, again use the Pythagorean Theorem to solve for BC:

    62 + 122 = (BC)2

    180 = (BC)2

    BC = \(\sqrt{180}\) = \(\sqrt{(36)(5)}\) = 6√5

If 93 − x = 342, then x =?

  • Solution

    Solve for x, and keep track of the negative signs.

    93 − x = 342

    −x = 342 − 93

    −x = 249

    x = −249

The graph below represents which of the following inequalities?

  • Solution

    Since the circle at −1 is closed, that means that −1 should be included.

    Eliminate answer choices A, B, and E, which do not include –1.

    Since the graph goes to the right, the inequality must be greater than or equal to −1.

Attempted

0

Correct

0

Wrong

0

Complete
Math Solved Practice Test
Attempted
Wrong
Correct