G is the sequence of numbers g1, g2, g3…gn such that each term following the first is one more than two times the preceding term. If g2 + g4 = 30 , what is the first term in the sequence?
-
Solution
It’s an algebra question with numbers for answer choices, so set up your scratch paper to Plug In the Answers. Start with choice (C); if the first term is 3⁄2, then one more than twice the previous term is 4, so that’s g2 .The sequence becomes easy to compute from there: g3 = 4 × 2 + 1 = 9; g4 = 9 × 2 + 1 = 19; and g2 + g4 = 4 + 19 = 23.That’s too small, so eliminate choices (C), (B), and (A). Between choices (D) and (E), it’s easier to double choice (E), so try that next: If g1 = , then g2 = 8, g3 = 17, and g4 = 35; now g2 + g4 = 8 + 35 = 41.This is too big, so eliminate choice (E) and select choice (D).
12x + 3 − (4 − 4x)
The above expression will equal an integer if x equals all of the following EXCEPT
Indicate all possible values.
-
Solution
A and D
If you simplify the expression you get 16x − 1. Now, plug in each of the answer choices.Choices (B) and (C) yield integer values, but choices (A) and (D) don’t.This is an interesting new feature of the new question types: Normally the EXCEPT/NOT/LEAST questions will have only one answer that doesn’t work. With ATA (All That Apply) questions, you can have multiple answers that don’t work. Be careful!
In a certain game, players have three chances during each turn to earn points. Each consecutive win awards more points than the previous win. The second win awards 100 points more than the ?rst, and the third win awards twice as many points as the second win. Tammy won the maximum number of points during her turn and received a total of 700 points. How many points are awarded for the first win?
-
Solution
With actual values in the answer choices, this question is a good example of when to use PITA. Set up columns with the first, second, and third wins labeled at the top.The correct answer should have the three amounts totaling 700. When you work through choice (C), you’ll see that it works—which means there’s no need to try any others.

In Seattle, the total rainfall in a certain year was 37 inches. From April to October of that year, the average rainfall was approximately 1.7 inches per month. What was the average rainfall, in inches, for the remaining months of the year?
-
Solution
Simplify this question by using Average Pies to deal with the two steps of the problem. First, use the pie on the left to figure out how many total inches of rain fell in the 7 months from April to October; you’ll get 11.9, which you can round up to 12. Next, subtract that total from 37 to find out how many remaining inches fell during the other 5 months. Finally, take those remaining 25 inches and divide by the remaining five months—as shown in the Average Pie on the right—and you’ll have choice (D).

Betty sold 3⁄5 of her family’s garage sale items. Ernest sold 1⁄2 of the remaining items. What fraction of the family’s garage sale items did Ernest sell?
-
Solution
1⁄5
Plug in a number for the original number of garage sale items that works with the fractions in the problem. It’s often easiest to multiply the denominators of all of the fractions in the problem. So let’s say there are 10 items.That means that Betty sold 6, and Ernest sold half of 4, or 2.The question asked for the fraction of items Ernest sold, and you solved for the number he sold.That’s okay, because all you have to do is put that number over the total number of items, 10. So,Ernest sold \(\frac{2}{10}\), or 1⁄5.
If the perimeter of a rectangle is 24 and the length is twice the width, what is the length of the rectangle?
-
Solution
Use Plugging In the Answers. Start with choice (C): If the length is 6, then the width is 3, and the perimeter is 18.That’s too small, so eliminate choices (A), (B), and (C). Go to choice (D). If the length of the rectangle is 8, then the width is 4, and the perimeter is 24. That matches the information from the question, so there’s no need for you to check choice (E).
If a + b = 15 and 2a − b = 6, what is the value of b?
-
Solution
Although you could solve the system of equations, it is easier and more reliable to plug in the answers. Since it is the middle value of this range of answers, begin by plugging in choice (C). If b = 8, then a must be equal to 7.Then, plug that into the second equation: 2 × 7 = 14. 14 − 8 = 6, which is what it was supposed to equal.The answer is choice (C).
A model rocket takes o/ from an elevated launch pad 9 feet above the ground. The rocket’s elevation follows the function h = −2t2 + 12t + 9, where h represents the height after t seconds. Which of the following ordered pairs (t, h) could be the rocket’s height at a given time?
Indicate all possible values.
-
Solution
A, D,E, and F
To solve this question, try Plugging In the Answers. For answer (A), if you plug t = 1 into the function, then h = 19, so choice (A) works. If t = 2.5, though, then h = 26.5, so eliminate choice (B). If t = 3, then h = 27, so eliminate choice (C). If t = 4, then h = 25, so choice (D) works. If t = 5.5, then h = 14.5, so choice (E) works. If t = 6, then h = 9, so choice (F) works. If t = 7, finally, then h = −5, which does not equal 1, so eliminate choice (G).Choices (A), (D), (E), and (F) are correct.
Shares of XYZ Co and ABC Corp are sold on a stock exchange that closes at 5 p.m. each day. At the close of business on Monday, shares of XYZ Co sold for half the price of shares of ABC Corp. On Tuesday and Wednesday, the price of shares of XYZ Co decreased at a rate of 1⁄5 per day. During that same period, shares of ABC Corp increased at a rate of sup>1⁄4 per day. At the close of business on Wednesday, the price of shares of ABC Corp was how many times greater, rounded to the nearest integer, than the price of shares of XYZ Co?
-
Solution
5
To solve this question, Plug In. Here, the “hidden” variable is the starting share price of either stock. A nice number to plug in will be divisible by 2, 4, and 5, such as 400. If XYZ Co sold on Monday for $400, then ABC Corp sold for $800.To calculate the price of XYZ Co on the next 2 days,1⁄5 of 400 is 80, so the price on Tuesday was $400 − $80 = $320;1⁄5 of 320 is 64, so the price at the close of business on Wednesday was $320 − $64 = $256. For ABC Corp,1⁄4 of 800 is 200, so the price on Tuesday was $800 + $200 = $1000;1⁄4 of 1000 is 250, so the price at the close of business on Wednesday was $1000 + $250 = $1250. Finally, divide the final price of ABC Corp by that of XYZ Co: 1250 ÷ 256 equals about 4.88, which rounds to 5.
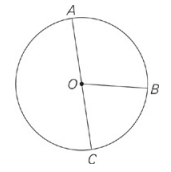
Note: Figure not drawn to scale
The radius of Circle Q is 8. If length of arc ABC is greater than 26, which of the following could be the value of the sums of angles AQB and BQC?
Indicate all possible values.
-
Solution
F and G
Plug in the answers. Normally, you’d start with one of the middle answer choices, but choice (E) looks easiest to solve. It’s half the circle; the circumference of the whole circle would be 16π, which can be approximated to about 50 using your on-screen calculator Half the circle, then, would be about 25. Since the length of the arc is greater than 26, choice (E) is too small, as are choices (A), (B), (C), and (D).Eliminate them all.Choice (F) makes the sum of the angles represent \(\frac{240}{360}\), or 2⁄3, of the whole circle;2⁄3 of about 50 is easily greater than 26, so choice (F) works, as does choice (G).

