refer to the following information.
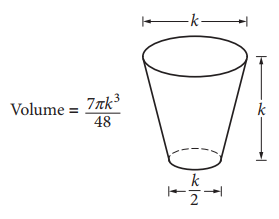
The glass pictured above can hold a maximum volume of 473 cubic centimeters, which is approximately 16 fluid ounces.
Water pours into the glass slowly and at a constant rate. Which of the following graphs best illustrates the height of the water level in the glass as it fills?
-
Solution
Due to the shape of the glass, if the water is poured at a constant rate, the height of the water level will increase faster initially, where the diameter of the glass is smaller, and increase more slowly later, as the diameter of the glass increases. Choice C is the only graph that shows this behavior: it is steeper initially and then gets less steep.
Choice A is incorrect since it shows the height of the water level increasing at a constant rate over time. Choice B is incorrect since it shows the height of the water level increasing slowly at first and faster later. Choice D is incorrect since it shows the height of the water level staying constant even as water is being poured into the glass.
refer to the following information.

The glass pictured above can hold a maximum volume of 473 cubic centimeters, which is approximately 16 fluid ounces.
What is the value of k, in centimeters?
-
Solution
Using the volume formula V = \(V=\frac{7πk^{3}}{48}\) and the give information that the volume of the glass is 473 cubic centimeters, the value of k can be found as follows:
\(473=\frac{7\pi k^{3}}{48}\)
\(K^{3}=\frac{473(48)}{7\pi }\)
\(K=\sqrt[3]{\frac{3473(48)}{7\pi} }\approx 10.10690\)
Therefore, the value of k is approximately 10.11 centimeters.
Choices A, B, and C are incorrect. Substituting the values of k from these choices in the formula results in volumes of approximately 7 cubic centimeters, 207 cubic centimeters, and 217 cubic centimeters, respectively, all of which contradict the given information that the volume of the glass is 473 cubic centimeters.
x + 1 = \(\frac{2}{x+1}\)
In the equation above, which of the following is a possible value of x + 1 ?
-
Solution
Multiplying both sides of the equation by x + 1 gives (x + 1)2 = 2. This means x + 1 is a number whose square is 2, so (x + 1) is either √2 or −√2 . Therefore, √2 is a possible value for x + 1.
Choice A is incorrect and may result from trying to find the value of x instead of x + 1 and making a sign error. Choice C is incorrect and may result from solving for (x + 1)2 instead of x + 1. Choice D is incorrect and may result from squaring instead of taking the square root to find the value of x + 1.
In the xy-plane, the graph of which of the following equations is a line with a slope of 3 ?
-
Solution
The graph of an equation given in the form y = mx + b has slope m. The equation in choice C is y = 3x + 2, so the slope of its graph is 3.
Choices A, B, and D are incorrect. They are all given in the form y = mx + b, where m is the slope. Therefore, choice A has a graph with a slope of 1⁄3 , choice B has a graph with a slope of 1 (because x = 1 ∙ x), and choice D has a graph with a slope of 6.
Two types of tickets were sold for a concert held at an amphitheater. Tickets to sit on a bench during the concert cost $75 each, and tickets to sit on the lawn during the concert cost $40 each. Organizers of the concert announced that 350 tickets had been sold and that $19,250 had been raised through ticket sales alone. Which of the following systems of equations could be used to find the number of tickets for bench seats, B, and the number of tickets for lawn seats, L, that were sold for the concert?
-
Solution
Since only two types of tickets were sold and a total of 350 tickets were sold, the sum of the numbers of both types of ticket sold must be 350. Therefore, B + L = 350. Since the bench tickets were $75 each, the income from B bench tickets was 75B. Similarly,since the lawn tickets were $40 each, the income from L lawn tickets sold was 40L. The total income from all tickets was $19,250. So the sum of the income from bench tickets and lawn tickets sold must equal 19,250. Therefore, 75B + 40L = 19,250. Only choice D has both correct equations.
Choice A is incorrect and may result from incorrectly multiplying the income from each type of ticket instead of adding them. It also incorrectly uses 1,950 instead of 19,250. Choice B is incorrect and may result from confusing the cost of bench tickets with the cost of lawn tickets. Choice C is incorrect and may result from confusing the total number of tickets sold with the total amount raised
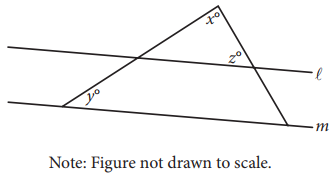
In the figure above, lines A and m are parallel, y = 20, and z = 60. What is the value of x ?
-
Solution
Let the measure of the third angle in the smaller triangle be a°. Since lines ℓ and m are parallel and cut by transversals, it follows that the corresponding angles formed are congruent. So a° = y° = 20°. The sum of the measures of the interior angles of a triangle is 180°, which for the interior angles in the smaller triangle yields a + x + z = 180. Given that z = 60 and a = 20, it follows that 20 + x + 60 = 180. Solving for x gives x = 180 − 60 − 20, or x = 100.
Choice A is incorrect and may result from incorrectly assuming that angles x + z = 180. Choice C is incorrect and may result from incorrectly assuming that the smaller triangle is a right triangle, with x as the right angle. Choice D is incorrect and may result from a misunderstanding of the exterior angle theorem and incorrectly assuming that x = y + z
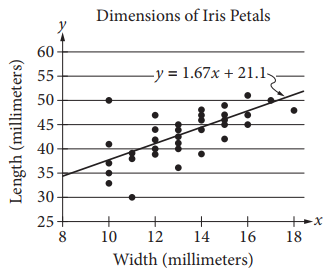
The scatterplot above shows data collected on the lengths and widths of Iris setosa petals. A line of best fit for the data is also shown. Based on the line of best fit, if the width of an Iris setosa petal is 19 millimeters, what is the predicted length, in millimeters, of the petal?
-
Solution
The given line of best fit can be used to predict the length when the width is known. The equation of the line of best fit is given as y = 1.67x + 21.1, where x is the width in millimeters and y is the predicted length in millimeters. If the width of the petal is 19 millimeters, then x = 19 and y = 1.67(19) + 21.1 = 52.83.
Choice A is incorrect and may result from incorrectly using x = 0 in the equation. Choice B is incorrect and may result from neglecting to add 21.1 in the computation. Choice D is incorrect and may result from an arithmetic error.
In a random sample of 200 cars of a particular model, 3 have a manufacturing defect. At this rate, how many of 10,000 cars of the same model will have a manufacturing defect?
-
Solution
The fraction of the cars in the random sample that have a manufacturing defect is \(\frac{3}{200}\) = 0.015. At this rate, out of 10,000 cars there would be 0.015 × 10,000 = 150 cars that have a manufacturing defect.
Choices B, C, and D are incorrect because the fractions of cars in the population that have a defect,\(\frac{200}{10,000}\) = 0.02 in choice B,\(\frac{250}{10,000}\) = 0.025 in choice C, and \(\frac{300}{10,000}\) = 0.03 in choice D, are all different from the fraction of cars in the sample with a manufacturing defect, which is 0.015.
Tracy collects, sells, and trades figurines, and she tracks the number of figurines in her collection on the graph below.

On what interval did the number of figurines decrease the fastest?
-
Solution
According to the graph, the number of figurines decreased between 1 and 2 months and between 3 and 4 months. Because the line segment between 3 and 4 months is steeper than the line segment between 1 and 2 months, it follows that the number of figurines decreased the fastest between 3 and 4 months.
Choice A is incorrect. Between 1 and 2 months, the number of figurines decreased. However, the number of figurines decreased faster during the interval between 3 and 4 months. Choices B and D are incorrect. The number of figurines during these intervals was increasing, not decreasing.
One pound of grapes costs $2. At this rate, how many dollars will c pounds of grapes cost?
-
Solution
If one pound of grapes costs $2, two pounds of grapes will cost 2 times $2, three pounds of grapes will cost 3 times $2, and so on. Therefore, c pounds of grapes will cost c times $2, which is 2c dollars.
Choice B is incorrect and may result from incorrectly adding instead of multiplying. Choice C is incorrect and may result from assuming that c pounds cost $2, and then finding the cost per pound. Choice D is incorrect and could result from incorrectly assuming that 2 pounds cost $c, and then finding the cost per pound.