The average annual energy cost for a certain home is $4,334. The homeowner plans to spend $25,000 to install a geothermal heating system. The homeowner estimates that the average annual energy cost will then be $2,712. Which of the following inequalities can be solved to find t, the number of years after installation at which the total amount of energy cost savings will exceed the installation cost?

25,000 > (4,334 − 2,712)t
25,000 < (4,334 − 2,712)t
25,000 − 4,334 > 2,712t
25,000 > $\frac{4,332}{2,712}$t

Solution

The savings each year from installing the geothermal heating system will be the average annual energy cost for the home before the geothermal heating system installation minus the average annual energy cost after the geothermal heating system installation, which is (4,334 − 2,712) dollars. In t years, the savings will be (4,334 − 2,712)t dollars. Therefore, the inequality that can be solved to find the number of years after installation at which the total amount of energy cost savings will exceed (be greater than) the installation cost, $25,000, is 25,000 < (4,334 − 2,712)t.

Choice A is incorrect. It gives the number of years after installation at which the total amount of energy cost savings will be less than the installation cost. Choice C is incorrect and may result from subtracting the average annual energy cost for the home from the onetime cost of the geothermal heating system installation. To find the predicted total savings, the predicted average cost should be subtracted from the average annual energy cost before the installation, and the result should be multiplied by the number of years, t. Choice D is incorrect and may result from misunderstanding the context. The ratio $\frac{4,332}{2,712}$ compares the average energy cost before installation and the average energy cost after installation; it does not represent the savings.

The range of the polynomial function f is the set of real numbers less than or equal to 4. If the zeros of f are −3 and 1, which of the following could be the graph of y = f(x) in the xy-plane?

Solution

Since zeros of f correspond to the x-intercepts of the graph of f, and the range of f gives all the possible y-values on the graph of the function, the correct graph of the function has only points with y-values less than or equal to 4, and crosses the x-axis at only (−3, 0) and (1, 0). The graph in choice A satisfies both of these conditions.

Choice B is incorrect. The graph of the function matches the range given, but the zeros are at −1 and 3, not −3 and 1. Choice C is incorrect. The graph has y-values greater than 4. Choice D is incorrect. Even though the graph has zeros at −3 and 1, it has an additional zero at 0, and the range of the graph is the set of all real numbers.

The figure below shows the relationship between the percent of leaf litter mass remaining after decomposing for 3 years and the mean annual temperature, in degrees Celsius (°C), in 18 forests in Canada. A line of best fit is also shown.

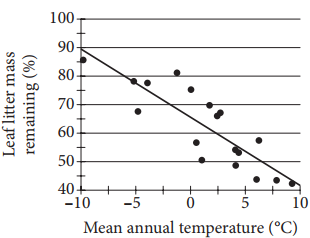
A particular forest in Canada, whose data is not included in the figure, had a mean annual temperature of −2°C. Based on the line of best fit, which of the following is closest to the predicted percent of leaf litter mass remaining in this particular forest after decomposing for 3 years?

Solution

Based on the line of best fit shown, the predicted percent of leaf litter mass remaining for a forest with a mean annual temperature of −2°C is about 70%.

Choice A is incorrect; it is the predicted percent of leaf litter mass remaining at about 6.5°C. Choice B is incorrect; it is the predicted percent of leaf litter mass remaining at 2°C instead of at −2°C.

Choice D is incorrect; it is the predicted percent of leaf litter mass remaining at about −7°C.

Ages of 20 Students Enrolled in a College Class

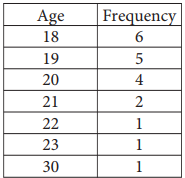
The table above shows the distribution of ages of the 20 students enrolled in a college class. Which of the following gives the correct order of the mean, median, and mode of the ages?

mode < median < mean
mode < mean < median
median < mode < mean
mean < mode < median

Solution

The mode is the data value with the highest frequency. So for the data shown, the mode is 18. The median is the middle data value when the data values are sorted from least to greatest. Since there are 20 ages ordered, the median is the average of the two middle values, the 10th and 11th, which for these data are both 19. Therefore, the median is 19. The mean is the sum of the data values divided by the number of the data values. So for these data, the mean is $\frac{(18 \times 6) + (19 \times 5) + (20 \times 4) + (21 \times 2) + (22 \times 1) + (23 \times 1) + (30 \times 1)}{20}$= 20.

Since the mode is 18, the median is 19, and the mean is 20, mode < median < mean.

Choice B and D are incorrect because the mean is greater than the median. Choice C is incorrect because the median is greater than the mode.

Alternate approach: After determining the mode, 18, and the median, 19, it remains to determine whether the mean is less than 19 or more than 19. Because the mean is a balancing point, there is as much deviation below the mean as above the mean. It is possible to compare the data to 19 to determine the balance of deviation above and below the mean. There is a total deviation of only 6 below 19 (the 6 values of 18); however, the data value 30 alone deviates by 11 above 19. Thus the mean must be greater than 19.

Number of Adults Contracting Colds

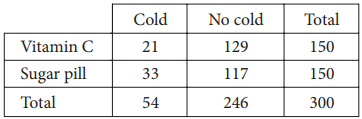
The table shows the results of a research study that investigated the therapeutic value of vitamin C in preventing colds. A random sample of 300 adults received either a vitamin C pill or a sugar pill each day during a 2-week period, and the adults reported whether they contracted a cold during that time period. What proportion of adults who received a sugar pill reported contracting a cold?

$\frac{11}{18}$
$\frac{11}{50}$
$\frac{9}{50}$
$\frac{11}{100}$

Solution

A total of 150 adults received the sugar pill. Of those, 33 reported contracting a cold. Therefore,$\frac{30}{150}$, or the equivalent $\frac{11}{50}$ , is the proportion of adults receiving a sugar pill who reported contracting a cold.

Choice A is incorrect. This is the proportion of adults receiving a sugar pill and contracting a cold to all adults contracting a cold ($\frac{33}{54}$).Choice C is incorrect. This is the proportion of adults who reported contracting a cold to all the participants in the study ($\frac{54}{300}=\frac{9}{50}$). Choice D is incorrect. This is the proportion of adults who received a sugar pill and reported contracting a cold to all the participants in the study ($\frac{54}{300}=\frac{11}{100}$).

A granite block in the shape of a right rectangular prism has dimensions 30 centimeters by 40 centimeters by 50 centimeters. The block has a density of 2.8 grams per cubic centimeter. What is the mass of the block, in grams? (Density is mass per unit volume.)

336
3,360
16,800
168,000

Solution

Since density is mass per unit volume, the mass is the density times volume. The volume of a right rectangular prism is the product of the lengths of the sides. Therefore:

mass = (2.8 grams per cubic centimeter) × (30 centimeters × 40 centimeters × 50 centimeters)

mass = (2.8 grams per cubic centimeter) × (60,000 cubic centimeters)

mass = 168,000 grams

Choice A is incorrect and may result from adding, instead of multiplying, the lengths of the sides to find the volume. Choice B is incorrect and may result from the same error as in choice A, as well as a place value error. Choice C is incorrect and may result from a place value error when finding the volume.

Which of the following is a value of x for which the expression $\frac{−3}{x^{2}+3x−10}$ is undefined?

−3
−2
zero
2

Solution

A rational expression is undefined when the denominator is 0. To determine the values of x that result in a denominator of 0, set the denominator equal to 0 and solve for x:

x² + 3x − 10 = 0

(x + 5)(x − 2) = 0

x + 5 = 0 or x − 2 = 0

x = −5 or x = 2

Among the answer choices, only the value x = 2 is listed, so choice D is correct.

Choice A is incorrect.When x = −3, the denominator is (−3)² + 3(−3) − 10 = −10, so the given expression is not undefined. Choice B is incorrect and may result from incorrectly factoring the denominator or incorrectly assuming that if (x − 2) is a factor, then x = −2 is a solution. Choice C is incorrect and may result from giving the value of the denominator that makes the given expression undefined rather than the value of x that makes the denominator equal to 0.

If a^–¹⁄₂ = x, where a > 0, what is a in terms of x ?

√x
−√x
¹⁄_x²
− ¹⁄_x²

Solution

Since a has the exponent ¹⁄₂, a can be isolated by raising both sides of the equation to the −2 power.

a ^{(−¹⁄₂)(−2)}

a = x^-2

a = x¹⁄_x²

Alternate method:

a^-¹⁄₂ = ¹⁄_a¹⁄₂

So,

¹⁄_√a = x

Square both sides of the equation:

¹⁄_a  = x²

Then take the reciprocal of both sides:

a = ¹⁄_x²

Choice A is incorrect and may result from incorrectly taking the square root of both sides to eliminate the exponent of a. Choice B is incorrect and may result from incorrectly taking the square root of both sides to eliminate the exponent of a, and incorrectly multiplying by −1 to make the exponent positive. Choice D is incorrect and may result from incorrectly multiplying by −1 to make the exponent positive.

Roberto is an insurance agent who sells two types of policies: a $50,000 policy and a $100,000 policy. Last month, his goal was to sell at least 57 insurance policies. While he did not meet his goal, the total value of the policies he sold was over $3,000,000. Which of the following systems of inequalities describes x, the possible number of $50,000 policies, and y, the possible number of $100,000 policies, that Roberto sold last month?

x + y < 57 50,000x + 100,000y < 3,000,000
x + y > 57 50,000x + 100,000y > 3,000,000
x + y < 57 50,000x + 100,000y > 3,000,000
x + y > 57 50,000 + 100,000 < 3,000,000

Solution

Since Roberto sells only two types of policies and he didn’t meet his goal of selling at least 57 policies, the sum of x, the number of $50,000 policies, and y, the number of $100,000 policies, must be less than 57. Symbolically, that is x + y < 57. The total value, in dollars, from selling x number of $50,000 policies is 50,000x. The total value, in dollars, from selling y number of $100,000 policies is 100,000y. Since the total value of the policies he sold was over $3,000,000, it follows that 50,000x + 100,000y > 3,000,000. Only choice C has both correct inequalities.

Choice A is incorrect because the total value, in dollars, of the policies Roberto sold was greater than, not less than, 3,000,000. Choice B is incorrect because Roberto didn’t meet his goal, so x + y should be less than, not greater than, 57. Choice D is incorrect because both inequalities misrepresent the situation.

refer to the following information.

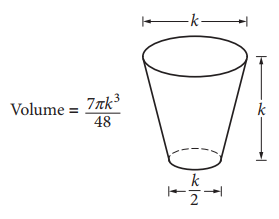
Jenny has a pitcher that contains 1 gallon of water. How many times could Jenny completely fill the glass with 1 gallon of water? (1 gallon = 128 fluid ounces)

Solution

It is given that the volume of the glass is approximately 16 fluid ounces. If Jenny has 1 gallon of water, which is 128 fluid ounces, she could fill the glass $\frac{128}{16}$ = 8 times.

Choice A is incorrect because Jenny would need 16 × 16 fluid ounces = 256 fluid ounces, or 2 gallons, of water to fill the glass 16 times. Choice C is incorrect because Jenny would need only 4 × 16 fluid ounces = 64 fluid ounces of water to fill the glass 4 times. Choice D is incorrect because Jenny would need only 3 × 16 fluid ounces = 48 fluid ounces to fill the glass 3 times.

UnAttempted

0

Attempted

0

Correct

0

Wrong

0

Complete