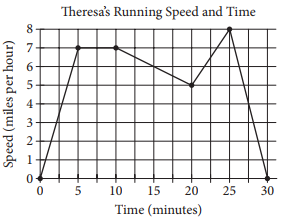
Theresa ran on a treadmill for thirty minutes, and her time and speed are shown on the graph above. According to the graph, which of the following statements is NOT true concerning Theresa’s run?

Theresa ran at a constant speed for five minutes.
Theresa’s speed was increasing for a longer period of time than it was decreasing.
Theresa’s speed decreased at a constant rate during the last five minutes.
Theresa’s speed reached its maximum during the last ten minutes.

Solution

Theresa’s speed was increasing from 0 to 5 minutes and from 20 to 25 minutes, which is a total of 10 minutes. Theresa’s speed was decreasing from 10 minutes to 20 minutes and from 25 to 30 minutes, which is a total of 15 minutes. Therefore, Theresa’s speed was NOT increasing for a longer period of time than it was decreasing.

Choice A is incorrect. Theresa ran at a constant speed for the 5-minute period from 5 to 10 minutes. Choice C is incorrect. Theresa’s speed decreased at a constant rate during the last 5 minutes. Choice D is incorrect. Theresa’s speed reached its maximum at 25 minutes, which is within the last 10 minutes.

A customer paid $53.00 for a jacket after a 6 percent sales tax was added. What was the price of the jacket before the sales tax was added?

$47.60
$50.00
$52.60
$52.84

Solution

Let x be the price, in dollars, of the jacket before sales tax. The price of the jacket after the 6% sales tax is added was $53. This can be expressed by the equation x + 0.06x = 53, or 1.06x = 53. Dividing each side of this equation by 1.06 gives x = 50. Therefore, the price of the jacket before sales tax was $50.

Choices A, C, and D are incorrect and may be the result of computation errors.

A software company is selling a new game in a standard edition and a collector’s edition. The box for the standard edition has a volume of 20 cubic inches, and the box for the collector’s edition has a volume of 30 cubic inches. The company receives an order for 75 copies of the game, and the total volume of the order to be shipped is 1,870 cubic inches. Which of the following systems of equations can be used to determine the number of standard edition games, s, and collector’s edition games, c, that were ordered?

75 − s = c 20s + 30c = 1,870
75 − s = c 30s + 20c = 1,870
s − c = 75 25(s + c) = 1,870
s − c = 75 30s + 20c = 1,870

Solution

The total number of copies of the game the company will ship is 75, so one equation in the system is s + c = 75, which can be written as 75 − s = c. Because each standard edition of the game has a volume of 20 cubic inches and s represents the number of standard edition games, the expression 20s represents the volume of the shipment that comes from standard edition copies of the game. Similarly, the expression 30c represents the volume of the shipment that comes from collector’s edition copies of the games. Because these volumes combined are 1,870 cubic inches, the equation 20s + 30c = 1,870 represents this situation. Therefore, the correct answer is choice A.

Choice B is incorrect. This equation gives the volume of each standard edition game as 30 cubic inches and the volume of each collector's edition game as 20 cubic inches. Choice C is incorrect. This is the result of finding the average volume of the two types of games, using that average volume (25) for both types of games, and assuming that there are 75 more standard editions of the game than there are collector’s editions of the game. Choice D is incorrect. This is the result of assuming that the volume of each standard edition game is 30 cubic inches, that the volume of each collector's edition game is 20 cubic inches, and that there are 75 more standard editions than there are collector’s editions.

Lani spent 15% of her 8-hour workday in meetings. How many minutes of her workday did she spend in meetings?

Solution

There are 60 minutes in one hour, so an 8-hour workday has (60)(8) = 480 minutes. To calculate 15% of 480, multiply 0.15 by 480: (0.15)(480) = 72. Therefore, Lani spent 72 minutes of her workday in meetings.

Choice A is incorrect because 1.2 is 15% of 8, which gives the time Lani spent of her workday in meetings in hours, not minutes. Choices B and C are incorrect and may be the result of computation errors.

9ax + 9b − 6 = 21

Based on the equation above, what is the value of ax + b ?

Solution

To isolate the terms that contain ax and b, 6 can be added to both sides of the equation, which gives 9ax + 9b = 27. Then, both sides of this equation can be divided by 9, which gives ax + b = 3.

Choices B, C, and D are incorrect and may result from computation errors.

refer to the following information.

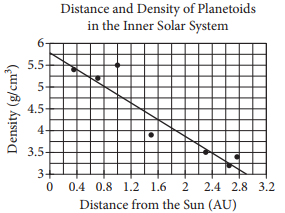
The scatterplot above shows the densities of 7 planetoids, in grams per cubic centimeter, with respect to their average distances from the Sun in astronomical units (AU). The line of best fit is also shown.

An astronomer has discovered a new planetoid about 1.2 AU from the Sun. According to the line of best fit, which of the following best approximates the density of the planetoid, in grams per cubic centimeter?

Solution

According to the line of best fit, a planetoid with a distance from the Sun of 1.2 AU has a density between 4.5 g/cm³ and 4.75 g/cm³.

The only choice in this range is 4.6. Choices A, B, and D are incorrect and may result from misreading the information in the scatterplot.

refer to the following information.

According to the scatterplot, which of the following statements is true about the relationship between a planetoid’s average distance from the Sun and its density?

Planetoids that are more distant from the Sun tend to have lesser densities.
Planetoids that are more distant from the Sun tend to have greater densities.
The density of a planetoid that is twice as far from the Sun as another planetoid is half the density of that other planetoid.
The distance from a planetoid to the Sun is unrelated to its density.

Solution

The slope of the line of best fit is negative, meaning as the distance of planetoids from the Sun increases, the density of the planetoids decreases. Therefore, planetoids that are more distant from the Sun tend to have lesser densities.

Choice B is incorrect because as the distance of planetoids from the sun increases, the density of the planetoids decreases. Choice C is incorrect. For example, according to the line of best fit, a planetoid that is 0.8 AU from the Sun has a density of 5 g/cm³, but a planetoid that is twice as far from the Sun with a distance of 1.6 AU has a density of 4.25 g/cm³. However, the density of 4.25 g/cm³ is not half the density of 5 g/cm³. Choice D is incorrect because there is a relationship between the distance from a planetoid to the Sun and density, as shown by the line of best fit.

Which of the following ordered pairs (x , y) satisfies the inequality 5x −3y < 4?

I. (1, 1)
II. (2, 5)
III. (3, 2)

I only
II only
I and II only
I and III only

Solution

Substituting (1, 1) into the inequality gives 5(1) − 3(1) < 4, or 2 < 4, which is a true statement. Substituting (2, 5) into the inequality gives 5(2) − 3(5) < 4, or −5 < 4, which is a true statement. Substituting (3, 2) into the inequality gives 5(3) − 3(2) < 4, or 9 < 4, which is not a true statement. Therefore, (1, 1) and (2, 5) are the only ordered pairs that satisfy the given inequality.

Choice A is incorrect because the ordered pair (2, 5) also satisfies the inequality. Choice B is incorrect because the ordered pair (1, 1) also satisfies the inequality. Choice D is incorrect because the ordered pair (3, 2) does not satisfy the inequality.

A market researcher selected 200 people at random from a group of people who indicated that they liked a certain book. The 200 people were shown a movie based on the book and then asked whether they liked or disliked the movie. Of those surveyed, 95% said they disliked the movie. Which of the following inferences can appropriately be drawn from this survey result?

At least 95% of people who go see movies will dislike this movie.
At least 95% of people who read books will dislike this movie.
Most people who dislike this book will like this movie.
Most people who like this book will dislike this movie.

Solution

The survey was given to a group of people who liked the book, and therefore, the survey results can be applied only to the population of people who liked the book. Choice D is the most appropriate inference from the survey results because it describes a conclusion about people who liked the book, and the results of the survey indicate that most people who like the book disliked the movie.

Choices A, B, and C are incorrect because none of these inferences can be drawn from the survey results. Choices A and B need not be true. The people surveyed all liked the book on which the movie was based, which is not true of all people who go see movies or all people who read books. Thus, the people surveyed are not representative of all people who go see movies or all people who read books. Therefore, the results of this survey cannot appropriately be extended to at least 95% of people who go see movies or to at least 95% of people who read books. Choice C need not be true because the sample includes only people who liked the book, and so the results do not extend to people who dislike the book.

A certain package requires 3 centimeters of tape to be closed securely. What is the maximum number of packages of this type that can be secured with 6 meters of tape? (1 meter = 100 cm)

Solution

Multiplying each side of 1 meter = 100 cm by 6 gives 6 meters = 600 cm. Each package requires 3 centimeters of tape. The number of packages that can be secured with 600 cm of tape is $\frac{600}{3}$, or 200 packages.

Choices A, B, and D are incorrect and may be the result of incorrect interpretations of the given information or of computation errors.

UnAttempted

0

Attempted

0

Correct

0

Wrong

0

Complete