Number of Registered Voters in the United States in 2012, in Thousands
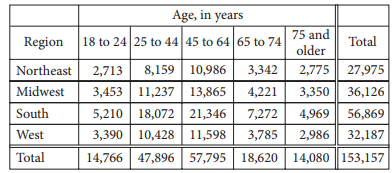
The table above shows the number of registered voters in 2012, in thousands, in four geographic regions and five age groups. Based on the table, if a registered voter who was 18 to 44 years old in 2012 is chosen at random, which of the following is closest to the probability that the registered voter was from the Midwest region?
-
Solution
According to the table, in 2012 there was a total of 14,766 + 47,896 = 62,662 registered voters between 18 and 44 years old, and 3,453 + 11,237 = 14,690 of them were from the Midwest region. Therefore, the probability that a randomly chosen registered voter who was between 18 and 44 years old in 2012 was from Midwest region is \(\frac{14,690}{62,662}\) ≈ 0.234. Of the given choices, 0.25 is closest to this value.
Choices A, C, and D are incorrect and may be the result of errors in selecting the correct proportion or in calculating the correct value.
Line A in the xy-plane contains points from each of Quadrants II, III, and IV, but no points from Quadrant I. Which of the following must be true?
-
Solution
The quadrants of the xy-plane are defined as follows: Quadrant I is above the x-axis and to the right of the y-axis; Quadrant II is above the x-axis and to the left of the y-axis; Quadrant III is below the x-axis and to the left of the y-axis; and Quadrant IV is below the x-axis and to the right of the y-axis. It is possible for line l to pass through Quadrants II, III, and IV, but not Quadrant I, only if line l has negative x- and y-intercepts. This implies that line l has a negative slope, since between the negative x-intercept and the negative y-intercept the value of x increases (from negative to zero) and the value of y decreases (from zero to negative); so the quotient of the change in y over the change in x, that is, the slope of line l, must be negative.
Choice A is incorrect because a line with an undefined slope is a vertical line, and if a vertical line passes through Quadrant IV, it must pass through Quadrant I as well. Choice B is incorrect because a line with a slope of zero is a horizontal line and, if a horizontal line passes through Quadrant II, it must pass through Quadrant I as well. Choice C is incorrect because if a line with a positive slope passes through Quadrant IV, it must pass through Quadrant I as well.
Movies with Greatest Ticket Sales in 2012
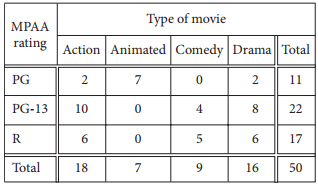
The table above represents the 50 movies that had the greatest ticket sales in 2012, categorized by movie type and Motion Picture Association of America (MPAA) rating. What proportion of the movies are comedies with a PG-13 rating?
-
Solution
According to the table, of the 50 movies with the greatest ticket sales in 2012, 4 are comedy movies with a PG-13 rating. Therefore, the proportion of the 50 movies with the greatest ticket sales in 2012 that are comedy movies with a PG-13 rating is \(\frac{4}{50}\), or equivalently, \(\frac{2}{25}\).
Choice B is incorrect; \(\frac{9}{50}\) is the proportion of the 50 movies with the greatest ticket sales in 2012 that are comedy movies, regardless of rating. Choice C is incorrect;\(\frac{9}{50}\) = \(\frac{4}{22}\) is the proportion of movies with a PG-13 rating that are comedy movies. Choice D is incorrect;\(\frac{11}{25}\) = \(\frac{22}{50}\) is the proportion of the 50 movies with the greatest ticket sales in 2012 that have a rating of PG-13.
Last week Raul worked 11 more hours than Angelica. If they worked a combined total of 59 hours, how many hours did Angelica work last week?
-
Solution
Let a be the number of hours Angelica worked last week. Since Raul worked 11 more hours than Angelica, Raul worked a + 11 hours last week. Since they worked a combined total of 59 hours, the equation a + (a + 11) = 59 must hold. This equation can be simplified to 2a + 11 = 59, or 2a = 48. Therefore, a = 24, and Angelica worked 24 hours last week. Choice B is incorrect because it is the number of hours Raul worked last week.
Choice C is incorrect. If Angelica worked 40 hours and Raul worked 11 hours more, Raul would have worked 51 hours, and the combined total number of hours they worked would be 91, not 59. Choice D is incorrect and may be the result of solving the equation a + 11 = 59 rather than a + (a + 11) = 59.
The density of an object is equal to the mass of the object divided by the volume of the object. What is the volume, in milliliters, of an object with a mass of 24 grams and a density of 3 grams per milliliter?
-
Solution
The density of an object is equal to the mass of the object divided by the volume of the object, which can be expressed as density =\(\frac{mass}{volume}\). Thus, if an object has a density of 3 grams per milliliter and a mass of 24 grams, the equation becomes 3 grams/milliliter =\(\frac{24\, grams}{volume}\).This can be rewritten as volume = \(\frac{24\, grams}{3\, grams/milliliter}\) = 8 milliliters.
Choice A is incorrect and be may be the result of confusing the density and the volume and setting up the density equation as 24 = \(\frac{3}{volume}\). Choice C is incorrect and may be the result of a conceptual error that leads to subtracting 3 from 24. Choice D is incorrect and may be the result of confusing the mass and the volume and setting up the density equation as 24 =\(\frac{volume}{3}\).
Nick surveyed a random sample of the freshman class of his high school to determine whether the Fall Festival should be held in October or November. Of the 90 students surveyed, 25.6% preferred October. Based on this information, about how many students in the entire 225-person class would be expected to prefer having the Fall Festival in October?
-
Solution
Because Nick surveyed a random sample of the freshman class, his sample was representative of the entire freshman class. Thus, the percent of students in the entire freshman class expected to prefer the Fall Festival in October is appropriately estimated by the percent of students who preferred it in the sample, 25.6%. Thus, of the 225 students in the freshman class, approximately 225 × 0.256 = 57.6 students would be expected to prefer having the Fall Festival in October. Of the choices given, this is closest to 60.
Choices A, C, and D are incorrect. These choices may be the result of misapplying the concept of percent or of calculation errors.
If a 3-pound pizza is sliced in half and each half is sliced into thirds, what is the weight, in ounces, of each of the slices? (1 pound = 16 ounces)
-
Solution
Because there are 16 ounces in 1 pound, a 3-pound pizza weighs 3 × 16 = 48 ounces. One half of the pizza weighs 1⁄2 × 48 = 24 ounces, and one-third of the half weighs 1⁄ × 24 = 8 ounces.
Alternatively, since 1⁄2 × 1⁄3 = 1⁄6, cutting the pizza into halves and then into thirds results in a pizza that is cut into sixths. Therefore, each slice of the 48-ounce pizza weighs 1⁄6 × 48 = 8 ounces.
Choice A is incorrect and is the result of cutting each half into sixths rather than thirds. Choice B is incorrect and is the result of cutting each half into fourths rather than thirds. Choice D is incorrect and is the result of cutting the whole pizza into thirds.
One of the requirements for becoming a court reporter is the ability to type 225 words per minute. Donald can currently type 180 words per minute, and believes that with practice he can increase his typing speed by 5 words per minute each month. Which of the following represents the number of words per minute that Donald believes he will be able to type m months from now?
-
Solution
Donald believes he can increase his typing speed by 5 words per minute each month. Therefore, in m months, he believes he can increase his typing speed by 5m words per minute. Because he is currently able to type at a speed of 180 words per minute, he believes that in m months, he will be able to increase his typing speed to 180 = 5m words per minute.
Choice A is incorrect because the expression indicates that Donald currently types 5 words per minute and will increase his typing speed by 180 words per minute each month. Choice B is incorrect because the expression indicates that Donald currently types 225 words per minute, not 180 words per minute. Choice D is incorrect because the expression indicates that Donald will decrease, not increase, his typing speed by 5 words per minute each month.
The monthly membership fee for an online television and movie service is $9.80. The cost of viewing television shows online is included in the membership fee, but there is an additional fee of $1.50 to rent each movie online. For one month, Jill’s membership and movie rental fees were $12.80. How many movies did Jill rent online that month?
-
Solution
Let m be the number of movies Jill rented online during the month. Since the monthly membership fee is $9.80 and there is an additional fee of $1.50 to rent each movie online, the total of the membership fee and the movie rental fees, in dollars, can be written as 9.80 + 1.50m. Since the total of these fees for the month was $12.80, the equation 9.80 + 1.50m = 12.80 must be true. Subtracting 9.80 from each side and then dividing each side by 1.50 yields m = 2.
Choices A, C, and D are incorrect and may be the result of errors in setting up or solving the equation that represents the context.
refer to the following information.
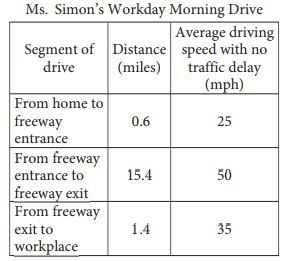
Ms. Simon drives her car from her home to her workplace every workday morning. The table above shows the distance, in miles, and her average driving speed, in miles per hour (mph), when there is no traffic delay, for each segment of her drive.
If Ms. Simon starts her drive at 6:30 a.m., she can drive at her average driving speed with no traffic delay for each segment of the drive. If she starts her drive at 7:00 a.m., the travel time from the freeway entrance to the freeway exit increases by 33% due to slower traffic, but the travel time for each of the other two segments of her drive does not change. Based on the table, how many more minutes does Ms. Simon take to arrive at her workplace if she starts her drive at 7:00 a.m. than if she starts her drive at 6:30 a.m.?
(Round your answer to the nearest minute.)
-
Solution
The correct answer is 6. Ms. Simon travels 15.4 miles on the freeway, and her average speed for this portion of the trip is 50 miles per hour when there is no traffic delay. Therefore, when there is no traffic delay, Ms. Simon spends \(\frac{15.4\, miles}{50\, mph}=0.308\, hours\) on the freeway. Since there are 60 minutes in one hour, she spends (0.308)(60) = 18.48 minutes on the freeway when there is no delay. Leaving at 7:00 a.m. results in a trip that is 33% longer, and 33% of 18.48 minutes is 6.16; the travel time for each of the other two segments does not change. Therefore, rounded to the nearest minute, it takes Ms. Simon 6 more minutes to drive to her workplace when she leaves at 7:00 a.m.