Of the following four types of savings account plans, which option would yield exponential growth of the money in the account?
-
Solution
Let I be the initial savings. If each successive year, 1% of the current value is added to the value of the account, then after 1 year, the amount in the account will be I + 0.01I = I(1 + 0.01); after 2 years, the amount in the account will be I(1 + 0.01) + 0.01I(1 + 0.01) = (1 + 0.01)I(1 + 0.01) = I(1 + 0.01)2; and after t years, the amount in the account will be I(1 + 0.01)t. This is exponential growth of the money in the account.
Choice A is incorrect. If each successive year, 2% of the initial savings, I, is added to the value of the account, then after t years, the amount in the account will be I + 0.02It, which is linear growth. Choice B is incorrect. If each successive year, 1.5% of the initial savings, I, and $100 is added to the value of the the account, then after t years the amount in the account will be I + (0.015I + 100)t, which is linear growth. Choice D is incorrect. If each successive year, $100 is added to the value of the account, then after t years the amount in the account will be I + 100t, which is linear growth.
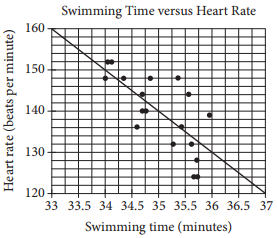
Michael swam 2,000 yards on each of eighteen days. The scatterplot above shows his swim time for and corresponding heart rate after each swim. The line of best fit for the data is also shown. For the swim that took 34 minutes, Michael’s actual heart rate was about how many beats per minutes less than the rate predicted by the line of best fit?
-
Solution
To answer this question, find the point in the graph that represents Michael’s 34-minute swim and then compare the actual heart rate for that swim with the expected heart rate as defined by the line of best fit. To find the point that represents Michael’s swim that took 34 minutes, look along the vertical line of the graph that is marked “34” on the horizontal axis. That vertical line intersects only one point in the scatterplot, at 148 beats per minute. On the other hand, the line of best fit intersects the vertical line representing 34 minutes at 150 beats per minute. Therefore, for the swim that took 34 minutes, Michael’s actual heart rate was 150 − 148 = 2 beats per minute less than predicted by the line of best fit.
Choices A, C, and D are incorrect and may be the result of misreading the scale of the graph.
Graphene, which is used in the manufacture of integrated circuits, is so thin that a sheet weighing one ounce can cover up to 7 football fields. If a football field has an area of approximately 11⁄3 acres,about how many acres could 48 ounces of graphene cover?
-
Solution
It is given that 1 ounce of graphene covers 7 football fields. Therefore, 48 ounces can cover 7 × 48 = 336 football fields. If each football field has an area of 11⁄3 acres, than 336 football fields have a total area of 336 × 11⁄3 = 448 acres. Therefore, of the choices given, 450 acres is closest to the number of acres 48 ounces of graphene could cover.
Choice A is incorrect and may be the result of dividing, instead of multiplying, the number of football fields by 11⁄3. Choice B is incorrect and may be the result of finding the number of football fields, not the number of acres, that can be covered by 48 ounces of graphene. Choice D is incorrect and may be the result of setting up the expression \(\frac{7 \times 48 \times 4}{3}\) and then finding only the numerator of the fraction.
refer to the following information.
S(P) = 1⁄2P + 40
D(P) = 220 − P
The quantity of a product supplied and the quantity of the product demanded in an economic market are functions of the price of the product. The functions above are the estimated supply and demand functions for a certain product. The function S(P) gives the quantity of the product supplied to the market when the price is P dollars, and the function D(P) gives the quantity of the product demanded by the market when the price is P dollars.
At what price will the quantity of the product supplied to the market equal the quantity of the product demanded by the market?
-
Solution
The quantity of the product supplied to the market will equal the quantity of the product demanded by the market if S(P) is equal to D(P), that is, if 1⁄2 P + 40 = 220 − P. Solving this equation gives P = 120, and so $120 is the price at which the quantity of the product supplied will equal the quantity of the product demanded.
Choices A, C, and D are incorrect. At these dollar amounts, the quantities given by S(P) and D(P) are not equal.
refer to the following information.
S(P) = 1⁄2P + 40
D(P) = 220 − P
The quantity of a product supplied and the quantity of the product demanded in an economic market are functions of the price of the product. The functions above are the estimated supply and demand functions for a certain product. The function S(P) gives the quantity of the product supplied to the market when the price is P dollars, and the function D(P) gives the quantity of the product demanded by the market when the price is P dollars.
How will the quantity of the product supplied to the market change if the price of the product is increased by $10 ?
-
Solution
The quantity of the product supplied to the market is given by the function S(P) = 1⁄2P + 40. If the price P of the product increases by $10, the effect on the quantity of the product supplied can be determined by substituting P + 10 for P as the argument in the function. This gives S(P + 10) = 1⁄2(P + 10) + 40 = 1⁄2P + 45, which shows that S(P + 10) = S(P) + 5. Therefore, the quantity supplied to the market will increase by 5 units when the price of the product is increased by $10.
Alternatively, look at the coefficient of P in the linear function S. This is the slope of the graph of the function, where P is on the horizontal axis and S(P) is on the vertical axis. Since the slope is 1⁄2, for every increase of 1 in P, there will be an increase of 1⁄2 in S(P), and therefore, an increase of 10 in P will yield an increase of 5 in S(P).
Choice A is incorrect. If the quantity supplied decreases as the price of the product increases, the function S(P) would be decreasing, but S(P) = 1⁄2P + 40 is an increasing function. Choice C is incorrect and may be the result of assuming the slope of the graph of S(P) is equal to 1. Choice D is incorrect and may be the result of confusing the y-intercept of the graph of S(P) with the slope, and then adding 10 to the y-intercept.
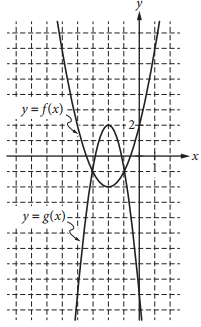
Graphs of the functions f and g are shown in the xy-plane above. For which of the following values of x does f(x)+ g(x)=0?
-
Solution
For any value of x, say x = x0, the point (x0 , f(x0)) lies on the graph of f and the point (x0, g(x0)) lies on the graph of g. Thus, for any value of x, say x = x0, the value of f(x0) + g(x0) is equal to the sum of the y-coordinates of the points on the graphs of f and g with x-coordinate equal to x0. Therefore, the value of x for which f(x) + g(x) is equal to 0 will occur when the y-coordinates of the points representing f(x) and g(x) at the same value of x are equidistant from the x-axis and are on opposite sides of the x-axis. Looking at the graphs, one can see that this occurs at x = −2: the point (−2, −2) lies on the graph of f, and the point (−2, 2) lies on the graph of g. Thus, at x = −2, the value of f(x) + g(x) is −2 + 2 = 0.
Choices A, C, and D are incorrect because none of these x-values satisfy the given equation, f(x) + g(x) = 0.
In order to determine if treatment X is successful in improving eyesight, a research study was conducted. From a large population of people with poor eyesight, 300 participants were selected at random. Half of the participants were randomly assigned to receive treatment X, and the other half did not receive treatment X. The resulting data showed that participants who received treatment X had significantly improved eyesight as compared to those who did not receive treatment X. Based on the design and results of the study, which of the following is an appropriate conclusion?
-
Solution
Experimental research is a method used to study a small group of people and generalize the results to a larger population. However, in order to make a generalization involving cause and effect:
⇒ The population must be well defined.
⇒ The participants must be selected at random.
⇒ The participants must be randomly assigned to treatment groups.
When these conditions are met, the results of the study can be generalized to the population with a conclusion about cause and effect. In this study, all conditions are met and the population from which the participants were selected are people with poor eyesight. Therefore, a general conclusion can be drawn about the effect of Treatment X on the population of people with poor eyesight.
Choice B is incorrect. The study did not include all available treatments, so no conclusion can be made about the relative effectiveness of all available treatments. Choice C is incorrect. The participants were selected at random from a large population of people with poor eyesight. Therefore, the results can be generalized only to that population and not to anyone in general. Also, the conclusion is too strong: an experimental study might show that people are likely to be helped by a treatment, but it cannot show that anyone who takes the treatment will be helped. Choice D is incorrect.This conclusion is too strong. The study shows that Treatment X is likely to improve the eyesight of people with poor eyesight, but it cannot show that the treatment definitely will cause improvement in eyesight for every person. Furthermore, since the people undergoing the treatment in the study were selected from people with poor eyesight, the results can be generalized only to this population, not to all people.
The cost of using a telephone in a hotel meeting room is $0.20 per minute. Which of the following equations represents the total cost c, in dollars, for h hours of phone use?
-
Solution
The hotel charges $0.20 per minute to use the meetingroom phone. This per-minute rate can be converted to the hourly rate using the conversion 1 hour = 60 minutes, as shown below.
\(\frac{\$ 0.20}{minute}\times \frac{60\, minutes}{1\, hour}=\frac{$(0.20\times 60)}{hour}\)
Thus, the hotel charges $(0.20 × 60) per hour to use the meeting-room phone. Therefore, the cost c, in dollars, for h hours of use is c = (0.20 × 60)h, which is equivalent to c = 0.20(60h).
Choice B is incorrect because in this expression the per-minute rate is multiplied by h, the number of hours of phone use. Furthermore, the equation indicates that there is a flat fee of $60 in addition to the per-minute or perhour rate. This is not the case. Choice C is incorrect because the expression indicates that the hotel charges $(\(\frac{60}{0.20}\)) per hour for use of the meetingroom phone, not $0.20(60) per hour. Choice D is incorrect because the expression indicates that the hourly rate is \(\frac{1}{60}\) times the per-minute rate, not 60 times the per-minute rate.
h = −16t2 + vt + k
The equation above gives the height h, in feet, of a ball t seconds after it is thrown straight up with an initial speed of v feet per second from a height of k feet. Which of the following gives v in terms of h, t, and k ?
-
Solution
Starting with the original equation, h = −16t2 + vt + k, in order to get v in terms of the other variables, −16t2 and k need to be subtracted from each side. This yields vt = h + 16t2 − k, which when divided by t will give v in terms of the other variables. However, the equation v = \(\frac{h+16t^{2}-k}{t}\) is not one of the options, so the right side needs to be further simplified. Another way to write the previous equation is v = \(\frac{h − k}{t}+\frac{16t^{2}}{t}\) , which can be simplified to v = \(\frac{h − k}{t}+16t\).
Choices A, B, and C are incorrect and may be the result of arithmetic errors when rewriting the original equation to express v in terms of h, t, and k.
If the function f has five distinct zeros, which of the following could represent the complete graph of f in the xy‑plane?
-
Solution
A zero of a function corresponds to an x-intercept of the graph of the function in the xy-plane. Therefore, the complete graph of the function f, which has five distinct zeros, must have five x-intercepts. Only the graph in choice D has five x-intercepts, and therefore, this is the only one of the given graphs that could be the complete graph of f in the xy-plane.
Choices A, B, and C are incorrect. The number of x-intercepts of each of these graphs is not equal to five; therefore, none of these graphs could be the complete graph of f, which has five distinct zeros.