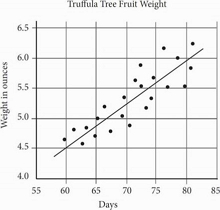
The scatterplot above shows the weight, in ounces, of the fruits on a certain truffula tree from days 55 to 85 after flowering. According to the line of best fit in the scatterplot above, which of the following is the closest approximation of the number of days after flowering of a truffula fruit that weighs 5.75 ounces?
-
Solution
Weight is shown on the vertical axis of the graph, given in ounces.
Make your own mark indicating 5.75 on this axis; then draw a horizontal line from that mark to the line of best fit.
Once you hit it, draw a vertical line straight down to the horizontal axis.
It should hit between 75 and 80 days, slightly closer to the mark for 75.
This makes (C) the credited response.
Draw your lines carefully, using your answer sheet as a straightedge if necessary.
Sai is ordering new shelving units for his store. Each unit is 7 feet in length and extends from floor to ceiling. Sai’s store has 119 feet of wall space that includes 21 feet of windows along the walls. If the shelving units cannot be placed in front of the windows, which of the following inequalities includes all possible values of r, the number of shelving units that Sai could use?
-
Solution
To figure out the total number of shelving units Sai could use, find the total available wall space and divide by the length of the units.
The total amount of wall space can be calculated as 119 - 21.
Because the length of each unit is 7 feet, the maximum number of units Sai could put up can be calculated as \(\frac{119 - 21}{7}\).
Because this is the maximum number of units Sai could put up, r has to be less than or equal to this number.
Therefore, the correct answer is (A).
If x2 + 12x = 64 and x > 0, what is the value of x ?
-
Solution
To solve the quadratic equation, first set the equation equal to 0.
The equation becomes x2 + 12x - 64 = 0. Next, factor the equation to get (x + 16)(x - 4) = 0.
Therefore, the two possible solutions for the quadratic equation are x + 16 = 0 and x - 4 = 0, so x = -16 or 4.
Since the question states that x > 0, x = 4 is the only possible solution.
Another way to approach this question is to plug in the answers.
Start with (B), x = 4. Plug 4 into the equation to get 42 + 12(4) = 64.
Solve the left side of the equation to get 16 + 48 = 64, or 64 = 64.
Since this is a true statement, the correct answer is (B).
The population, P, of Town Y since 1995 can be estimated by the equation P = 1.0635x + 3,250, where x is the number of years since 1995 and 0 ≤ x ≤ 20. In the context of this equation, what does the number 1.0635 most likely represent?
-
Solution
Use Process of Elimination to answer this question.
According to the question, P represents the population, so the outcome of the entire equation has something to do with the population.
Therefore, eliminate both (A) and (B) because 1.0635 can't represent the population if P does.
In the equation given, the only operations are multiplication and addition, which means that over time the population would increase.
Therefore, eliminate (D).
The correct answer is (C).
A toy pyramid (not shown) is made from poly(methyl methacrylate), better known by its trade term Lucite. The toy pyramid has a regular hexagonal base of 15 cm2 and a height of 4 cm. In the base of the pyramid, there is a semispherical indentation 2 cm in diameter. If the pyramid weighs 21.129 g, then what is the density of Lucite? (Density equals mass divided by volume.)
-
Solution
Work the problem in steps. You are given the mass, so to find density you need to find the volume of the pyramid.
The formula at the beginning of the section tells you that, for a pyramid, V = 1⁄3Bh, where B is the area of the base of the pyramid and h is the height.
Therefore, the volume of the pyramid is 1⁄3(15)(4) = 20.
However, you need to subtract the volume of the semispherical indentation in the base.
Once again, the reference sheet found beginning of the Math section tells you that the volume of a sphere is given by the equation V = 4⁄3πr3.
Because the diameter of the indentation is 2 cm, the radius of the hemisphere is 1 cm.
If it were a whole sphere, the volume of the indentation would be 4⁄3π(1)3 = 4.189; you want only half, so dividing by 2 gives you 2.094 cm3 for the hemisphere.
Subtracting 2.094 cm3 from the 20 cm3 of the pyramid gives you a total volume of 20 - 2.094 = 17.906 cm3.
Finally, you can find the density of Lucite by using the definition of density:
Density = \(\frac{21.129 \; g}{17.906 \; cm^{3}} \approx 1.18 \; g/cm^{3}\), which is (B).
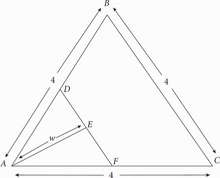
ΔABC is equilateral and ∠AEF is a right angle. D and F are the midpoints of AB and AC, respectively. What is the value of w ?
-
Solution
There's a lot going on in this problem! But if we take it piece by piece, we'll crack it.
Let's start filling in some information. The first thing the problem tells us is that triangle ABC is equilateral.
Mark 60 degree angles on the figure. Next, we see that angle AEF is a right angle.
Write that in as well.
The problem also conveniently tells us that D and F are the midpoints of AB and AC, respectively.
Therefore, AD and AF are 2.
Finally, the last piece of information reveals that E is the midpoint of DF; mark DE and EF as equal.
Now, what do we have? Triangle AEF is a right triangle, with a hypotenuse of 2 and a leg of 1.
Hmm, perhaps the good ol' Pythagorean theorem can help us.
Plug the numbers into the theorem, and you'll see that the answer is (B).
You may have also noticed that triangle ADE is a 30°-60°-90° triangle with hypotenuse 2, which means that DE is 1 and w, opposite the 60°, is the square root of 3.
In geometry questions on the SAT, there will often be multiple ways to get to the answer.
On the day of the test, use whichever way you are most comfortable with.
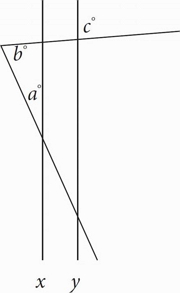
In the figure above, x || y. What is the value of a ?
-
Solution
Don't forget that you can plug in numbers on geometry questions.
Let's make b = 70° and a = 30°. So the third angle in the triangle is 80°.
You know that c would be 80°, because it is opposite an 80° angle.
Your target answer is a = 30°, so plug in 80° and 70° to find it.
The only possible answer is (D).
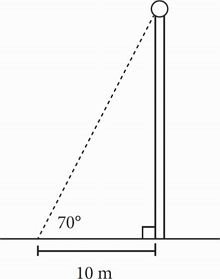
Martin wants to know how tall a certain flagpole is. Martin walks 10 meters from the flagpole, lies on the ground, and measures an angle of 70° from the ground to the base of the ball at the top of the flagpole. Approximately how tall is the flagpole from the ground to the base of the ball at the top of the flagpole?
-
Solution
Use SOHCAHTOA and your calculator to find the height of the flagpole.
From the 70° angle, you know the adjacent side of the triangle, and you want to find the opposite side, so you need to use tangent.
Tangent \(\frac{opposite}{adjacent}\), so tan 70° = \(\frac{x}{10m}\), where x is the height of the flagpole up to the ball.
Isolate x by multiplying both sides by 10: 10 tan 70° = x.
Use your calculator to find that 10 tan 70° = 27.47, which is closest to (C).

In the figure above, what is the length of \(\overline{BD}\)?
-
Solution
The 5 equal lengths that make up the two sides of the largest triangle tell us that we are dealing with 5 similar triangles.
The largest triangle has sides 15:25:30, and the sides of all 5 triangles will have an equivalent ratio.
Reduced, the ratio is 3:5:6, which happens to be the dimensions of the smallest triangle.
We want to find the length of BD, the base of a triangle with sides of 6 and 10.
This is twice as big as the smallest triangle, so the base BD must be 6 × 2 = 12, which is (C).
If a rectangular swimming pool has a volume of 16,500 cubic feet, a uniform depth of 10 feet, and a length of 75 feet, what is the width of the pool, in feet?
-
Solution
For this question, you need to know that volume equals length × width × height.
You know that the volume is 16,500, the depth (or height) is 10, and the length is 75.
Just put those numbers in the formula: 16,500 = 75 × w × 10.
Use your calculator to solve for w, which equals 22: (A).