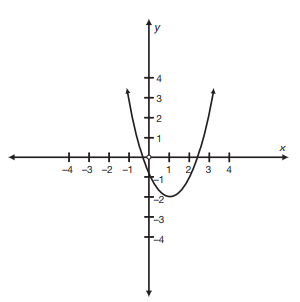
What is the equation of the parabola shown below?
-
Solution
The vertex of this parabola is at (1,–2). A parabola of the form y = (x + c)2 + d has its vertex at (–c,d). Therefore, the equation of a parabola whose vertex is (1,–2) is y= (x – 1)2 – 2. This parabola is similar to the parabola y = x2, but shifted right 1 unit and down 2 units.
Which of the following is the equation of a parabola whose vertex is (5,0)?
-
Solution
A parabola of the form y = (x + c)2 + d has its vertex at (–c,d). Therefore, the equation of a parabola whose vertex is (5,0) is y = (x – 5)2 + 0, or y = (x – 5)2. This parabola is similar to the parabola y = x2, but shifted right 5 units.
What is the vertex of the parabola whose equation is y = (x + 2)2 + 2?
-
Solution
A parabola of the form y = (x + c)2 + d has its vertex at (–c,d). Therefore, the vertex of the parabola whose equation is y = (x + 2)2 + 2 is (–2,2). This parabola is similar to the parabola y = x2, but shifted left 2 units and up 2 units.
Which of the following is the equation of a parabola whose vertex is (–3,–4)?
-
Solution
A parabola of the form y = (x + c)2 + d has its vertex at (–c,d). Therefore, the equation of a parabola whose vertex is (–3,–4) is y = (x + 3)2 – 4. This parabola is similar to the parabola y = x2, but shifted left 3 units and down 4 units.
What is the vertex of the parabola whose equation is y = x2 + 4?
-
Solution
A parabola of the form y = x2 + c has its vertex at (0,c). Therefore, the vertex of this parabola is at (0,4). This parabola is similar to the parabola y = x2, but shifted up 4 units.
What values make the fraction \(\frac{(x^{2}-36)}{(2x^{2}-25x+72)}\) undefined?
-
Solution
A fraction is undefined when its denominator is equal to 0. Factor the quadratic in the denominator and set each factor equal to 0 to find the values that make the fraction undefined. To find the factors of a quadratic, begin by finding two numbers whose product is equal to the constant of the quadratic. Since the first term of the quadratic is 2x2, the factors of the quadratic will be (2x + c)(x + d), where c and d are constants that multiply to 72 and add to –25 after either c or d is multiplied by 2. –8 and –9 multiply to 72, and 2(8) + 9 = 25. Therefore, the factors of 2x2 – 25x + 72 are (2x – 9) and (x – 8). Set each factor equal to 0 and solve for x: 2x – 9 = 0, 2x = 9, x = 9⁄2, and x – 8 = 0, x = 8. When x equals 9⁄2 or 8, the fraction is undefined.
What is one value that makes the fraction \(\frac{(x^{2})}{(9x^{2}-1)}\) undefined?
-
Solution
A fraction is undefined when its denominator is equal to 0. Set the denominator equal to 0 and solve for x; 9x2 – 1 = 0, 9x2 = 1, x2 = 1⁄9, x = – 1⁄3 or 1⁄3.
What is one value that makes the fraction \(\frac{(x-16)}{(x^{2}-16)}\) undefined?
-
Solution
A fraction is undefined when its denominator is equal to 0. Set the denominator equal to 0 and solve for x; x2 – 16 = 0, x2 = 16, x = –4 or 4.
What values make the fraction \(\frac{(x^{2}+8x+7)}{(x^{2}-8x+7)}\) undefined?
-
Solution
A fraction is undefined when its denominator is equal to 0. Factor the quadratic in the denominator and set each factor equal to 0 to find the values that make the fraction undefined. To find the factors of a quadratic, begin by finding two numbers whose product is equal to the constant of the quadratic. Of those numbers, find the pair that adds to the coefficient of the x term of the quadratic; –1 and –7 multiply to 7 and add to –8. Therefore, the factors of x2 – 8x + 7 are (x – 1) and (x – 7).Set each factor equal to 0 and solve for x: x – 1 = 0, x = 1, and x – 7 = 0, x = 7. When x equals 1 or 7, the fraction is undefined.
What is one value that makes the fraction \(\frac{(4x-8)}{(x^{2}+x-42)}\) undefined?
-
Solution
A fraction is undefined when its denominator is equal to 0. Factor the quadratic in the denominator and set each factor equal to 0 to find the values that make the fraction undefined. To find the factors of a quadratic, begin by finding two numbers whose product is equal to the constant of the quadratic. Of those numbers, find the pair that adds to the coefficient of the x term of the quadratic; –6 and 7
multiply to –42 and add to 1. Therefore, the factors of x2 + x – 42 are (x – 6) and (x + 7). Set each factor equal to 0 and solve for x: x – 6 = 0, x = 6, and x + 7 = 0, x = –7. When x equals 6 or –7, the fraction is undefined.