If angle 4 is congruent to angle 7, which of the following is NOT true?

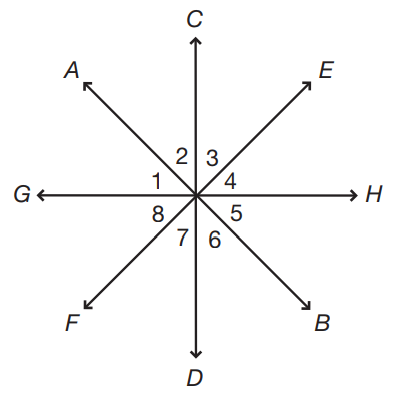
Lines CD and GH are perpendicular to each other, and every line goes through point O (not labeled). Every angle is greater than 0°.

Solution

Angles 3 and 7 are vertical angles, so their measures are equal. Angles 4 and 8 are also vertical angles, so their measures are also equal. Since angles 4 and 7 are congruent, and angles 4 and 8 are congruent, angles 7 and 8 must be congruent. In the same way, since angles 4 and 7 are congruent and angles 3 and 7 are congruent, angles 3 and 4 must be congruent. Angles 3, 4, 7, and 8 are all congruent. In fact, since angles 3 and 4 are complementary and angles 7 and 8 are complementary (since lines CD and GH are perpendicular) all four angles measure 45°. However, nothing is known about angles 1, 2, 5, and 6. It cannot be stated that angle 2 = angle 3.

If the measure of angle 1 is 3x + 5 and the measure of angle 6 is 5x – 3, what is the measure of angle 2?

Lines CD and GH are perpendicular to each other, and every line goes through point O (not labeled). Every angle is greater than 0°.

Solution

Angles 2 and 6 are vertical angles; their measures are equal. Therefore, the measure of angle 2 also 5x – 3. Since lines CD and GH are perpendicular to each other, angle COG is a right angle, and angles 1 and 2 are complementary; 3x + 5 + 5x – 3 = 90, 8x + 2 = 90, 8x = 88, x = 11. The measure of angle 2 is 5(11) – 3 = 55 – 3 = 52°.

If the sum of angles 2 and 3 is x² and the sum of angles 6 and 7 is 10x, what is the sum of angles 4 and 5?

Lines CD and GH are perpendicular to each other, and every line goes through point O (not labeled). Every angle is greater than 0°.

Solution

Angles 2 and 6 are vertical angles and angles 3 and 7 are vertical angles. Therefore, angle 2 is equal to angle 6, angle 3 is equal to angle 7, and the sum of angles 2 and 3 is equal to the sum of angles 6 and 7: x² = 10x; x² – 10x = 0, x(x – 10) = 0. x cannot be 0, since no angles measure 0°; x – 10 = 0, x = 10, and the sum of angles 2 and 3 is (10)² = 100. Since angles 2, 3, 4, and 5 form a line, the sum of their measures is 180°. Therefore, the sum of angles 4 and 5 is equal to 180 – 100 = 80°.

Which of the following number sentences is true?

Lines CD and GH are perpendicular to each other, and every line goes through point O (not labeled). Every angle is greater than 0°.

Solution

Angles 4 and 8 are vertical angles, angles 1 and 5 are vertical angles, and angles 2 and 6 are vertical angles. Since vertical angles have equal measures, the sum of angles 4, 5, and 6 is equal to the sum of angles 1, 2, and 8. Lines AB and EF are not known to be perpendicular. Test the other answer choices with different possible values. Angles 3 and 7 could measure 40°, while angles 1, 2, 5, and 6 measure 45°, and angles 4 and 8 measure 50°. Using these numbers, only the number sentence in choice e holds true.

If lines EF and AB are perpendicular, the measure of angle 4 is 11x, and the measure of angle 1 is 7x, what is the measure of angle 4?

Lines CD and GH are perpendicular to each other, and every line goes through point O (not labeled). Every angle is greater than 0°.

Solution

Since lines EF and AB are perpendicular, angle AOE measures 90°. Angle AOE is made up of angles 2 and 3. Angles 1, 2, 3, and 4 form a line. Since the measure of angles 2 and 3 add to 90°, and there are 180° in a line, the measures of angles 1 and 4 must add to 90°; 11x + 7x = 90, 18x = 90, x = 5. Therefore, the measure of angle 4 is 11(5) = 55°.

If angle JLB is three and a half times the size of angle LIF, what is the measure of angle EIL?

Lines EF and GH are parallel to each other and line IJ is perpendicular to lines EF and GH.

Solution

Angles JLB, ILH, and EIL are alternating angles; therefore, their measures are equal. Since angles EIL and LIF form a line, these angles are supplementary and add to 180°. If angle LIF is x, then angle JLB is 3.5x, and x + 3.5x = 180, 4.5x = 180, x = 40. Therefore, the measure of angle LIF is 40° and the measure of angle EIL is 3.5(40) = 140°.

If angles LIF and EIK are congruent, and the measure of angle LIF is 6 greater than the measure of angle JIL, what is the measure of angle KIJ?

Lines EF and GH are parallel to each other and line IJ is perpendicular to lines EF and GH.

Solution

Since line IJ is perpendicular to line EF, angles JIF and EIJ are right angles, which measures 90° each. Angles LIF and JIL combine to form angle JIF; therefore, their measures add to 90°. If the measure of angle JIL is x, then the measure of angle LIF is x + 6, since its measure is 6 greater than the measure of angle JIL; x + x + 6 = 90, 2x + 6 = 90, 2x = 84, x = 42. The measure of angle JIL is 42° and the measure of angle LIF is 42 + 6 = 48°. Since angles LIF and EIK are congruent, angle EIK is also 48°. Angles EIK and KIJ are complementary, so angle KIJ is 90 – 48 = 42°.

If the measure of angle GKI is 15x – 4 and the measure of angle CIF is x², what is the measure of angle EIC?

Lines EF and GH are parallel to each other and line IJ is perpendicular to lines EF and GH.

Solution

Angles GKI and EIC are alternating angles; therefore, angle EIC also measures 15x – 4. Since angles EIC and CIF form a line, their measures add to 180°; 15x – 4 + x2 = 180, x² + 15x – 184 = 0. Factor and solve for x: (x + 23)(x – 8) = 0, x + 23 = 0, x = –23. An angle cannot have a negative value, so disregard the negative value of x; x – 8 = 0, x = 8. Therefore, the measure of angle EIC is equal to: 15(8) – 4 = 120 – 4 = 116°.

If the measure of angle JLI is 8x – 4 and the measure of angle JIL is 5x + 3, what is the measure of angle AIE?

Lines EF and GH are parallel to each other and line IJ is perpendicular to lines EF and GH.

Solution

Since line IJ is perpendicular to line GH, angle IJL is a right angle, which measures 90°. There are 180° in a triangle, so the measures of angles JLI and JIL must add to 90°: 8x – 4 + 5x + 3 = 90, 13x – 1 = 90, 13x = 91, x = 7. Therefore, the measure of angle JLI is 8(7) – 4 = 52°. Since angles JLI and AIE are alternating angles, their measures are equal. Angle AIE is also 52°.

Which of the following pairs of angles must be congruent?

Lines EF and GH are parallel to each other and line IJ is perpendicular to lines EF and GH.

Solution

Since angles AIC and KIL are vertical angles, their measures are equal.

UnAttempted

0

Attempted

Correct

Wrong