refer to the following information.
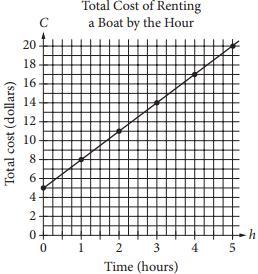
The graph above displays the total cost C, in dollars, of renting a boat for h hours.
What does the C-intercept represent in the graph?
-
Solution
The total cost C of renting a boat is the sum of the initial cost to rent the boat plus the product of the cost per hour and the number of hours, h, that the boat is rented. The C-intercept is the point on the C-axis where h, the number of hours the boat is rented, is 0. Therefore, the C-intercept is the initial cost of renting the boat.
Choice B is incorrect because the graph represents the cost of renting only one boat. Choice C is incorrect because the total number of hours of rental is represented by h-values, each of which corresponds to the first coordinate of a point on the graph. Choice D is incorrect because the increase in cost for each additional hour is given by the slope of the line, not by the C-intercept.
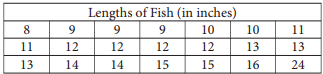
The table above lists the lengths, to the nearest inch, of a random sample of 21 brown bullhead fish. The outlier measurement of 24 inches is an error. Of the mean, median, and range of the values listed, which will change the most if the 24-inch measurement is removed from the data?
-
Solution
The range of the 21 fish is 24 − 8 = 16 inches, and the range of the 20 fish after the 24-inch measurement is removed is 16 − 8 = 8 inches. The change in range, 8 inches, is much greater than the change in the mean or median.
Choice A is incorrect. Let m be the mean of the lengths, in inches, of the 21 fish. Then the sum of the lengths, in inches, of the 21 fish is 21m. After the 24-inch measurement is removed, the sum of the lengths, in inches, of the remaining 20 fish is 21m − 24, and the mean length, in inches, of these 20 fish is \(\frac{21m − 24}{20}\) , which is a change of \(\frac{24 − m}{20}\) inches. Since m must be between the smallest and largest measurements of the 21 fish, it follows that 8 < m < 24, from which it can be seen that the change in the mean, in inches, is between \(\frac{24 − 24}{20}\) = 0 and \(\frac{24 − 8}{20}\) = 4⁄5, and so must be less than the change in the range, 8 inches. Choice B is incorrect because the median length of the 21 fish is the length of the 11th fish, 12 inches. After removing the 24-inch measurement, the median of the remaining 20 lengths is the average of the 10th and 11th fish, which would be unchanged at 12 inches. Choice D is incorrect because the changes in the mean, median, and range of the measurements are different.
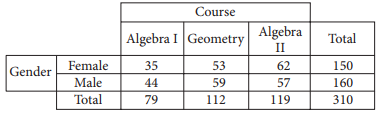
A group of tenth-grade students responded to a survey that asked which math course they were currently enrolled in. The survey data were broken down as shown in the table above. Which of the following categories accounts for approximately 19 percent of all the survey respondents?
-
Solution
From the table, there was a total of 310 survey respondents, and 19% of all survey respondents is equivalent to \(\frac{19}{100}\)× 310 = 58.9 respondents.Of the choices given, 59, the number of males taking geometry, is closest to 58.9 respondents.
Choices A, B, and D are incorrect because the number of males taking geometry is closer to 58.9 than the number of respondents in each of these categories.

Based on the histogram above, of the following, which is closest to the average (arithmetic mean) number of seeds per apple?
-
Solution
The average number of seeds per apple is the total number of seeds in the 12 apples divided by the number of apples, which is 12. On the graph, the horizontal axis is the number of seeds per apple and the height of each bar is the number of apples with the corresponding of seeds. The first bar on the left indicates that 2 apples have 3 seeds each, the second bar indicates that 4 apples have 5 seeds each, the third bar indicates that 1 apple has 6 seeds, the fourth bar indicates that 2 apples have 7 seeds each, and the fifth bar indicates that 3 apples have 9 seeds each. Thus, the total number of seeds for the 12 apples is (2 × 3) + (4 × 5) + (1 × 6) + (2 × 7) + (3 × 9) = 73, and the average number of seeds per apple is \(\frac{73}{12}\)= 6.08. Of the choices given, 6 is closest to 6.08.
Choice A is incorrect; it is the number of apples represented by the tallest bar but is not the average number of seeds for the 12 apples. Choice B is incorrect; it is the number of seeds per apple corresponding to the tallest bar, but is not the average number of seeds for the 12 apples. Choice D is incorrect; a student might choose this by correctly calculating the average number of seeds, 6.08, but incorrectly rounding up to 7.
Which of the following numbers is NOT a solution of the inequality 3x − 5 ≥ 4x − 3?
-
Solution
Subtracting 3x and adding 3 to both sides of 3x − 5 ≥ 4x − 3 gives −2 ≥ x. Therefore, x is a solution to 3x − 5 ≥ 4x − 3 if and only if x is less than or equal to −2 and x is NOT a solution to 3x − 5 ≥ 4x − 3 if and only if x is greater than −2. Of the choices given, only −1 is greater than −2 and, therefore, cannot be a value of x.
Choices B, C, and D are incorrect because each is a value of x that is less than or equal to −2 and, therefore, could be a solution to the inequality.
refer to the following information.
a = 1,052 + 1.08t
The speed of a sound wave in air depends on the air temperature. The formula above shows the relationship between a, the speed of a sound wave, in feet per second,and t, the air temperature, in degrees Fahrenheit (°F).
At which of the following air temperatures will the speed of a sound wave be closest to 1,000 feet per second?
-
Solution
Substituting 1,000 for a in the equation a = 1,052 + 1.08t gives 1,000 = 1,052 + 1.08t, and thus t = \(\frac{−52}{1.08}\) ≈ − 48.15. Of the choices given, −48°F is closest to −48.15°F. Since the equation a = 1,052 + 1.08t is linear, it follows that of the choices given, −48°F is the air temperature when the speed of a sound wave is closest to 1,000 feet per second.
Choices A, C, and D are incorrect, and might arise from errors in calculating \(\frac{−52}{1.08}\) or in rounding the result to the nearest integer. For example,choice C could be the result of rounding −48.15 to −49 instead of −48.
refer to the following information.
a = 1,052 + 1.08t
The speed of a sound wave in air depends on the air temperature. The formula above shows the relationship between a, the speed of a sound wave, in feet per second,and t, the air temperature, in degrees Fahrenheit (°F).
Which of the following expresses the air temperature in terms of the speed of a sound wave?
-
Solution
Subtracting 1,052 from both sides of the equation a = 1,052 + 1.08t gives a − 1,052 = 1.08t. Then dividing both sides of a − 1,052 = 1.08t by 1.08 gives t = \(\frac{a − 1,052}{1.08}\).
Choices B, C, and D are incorrect and could arise from errors in rewriting a = 1,052 + 1.08t. For example, choice B could result if 1,052 is added to the left side of a = 1,052 + 1.08t and subtracted from the right side, and then both sides are divided by 1.08.
For what value of n is |n − 1| + 1 equal to 0 ?
-
Solution
If the value of |n − 1| + 1 is equal to 0, then |n − 1| + 1 = 0. Subtracting 1 from both sides of this equation gives |n − 1| = −1. The expression |n − 1| on the left side of the equation is the absolute value of n − 1, and the absolute value can never be a negative number. Thus |n − 1| = −1 has no solution. Therefore, there are no values for n for which the value of |n − 1| + 1 is equal to 0.
Choice A is incorrect because |0 − 1| + 1 = 1 + 1 = 2, not 0. Choice B is incorrect because |1 − 1| + 1 = 0 + 1 = 1, not 0. Choice C is incorrect because |2 − 1| + 1 = 1 + 1 = 2, not 0.
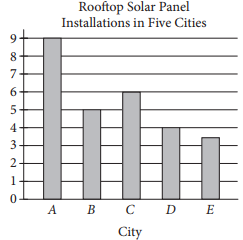
The number of rooftops with solar panel installations in 5 cities is shown in the graph above. If the total number of installations is 27,500, what is an appropriate label for the vertical axis of the graph?
-
Solution
Let x represent the number of installations that each unit on the y-axis represents. Then 9x, 5x, 6x, 4x, and 3.5x are the number of rooftops with solar panel installations in cities A, B, C, D, and E, respectively. Since the total number of rooftops is 27,500, it follows that 9x + 5x + 6x + 4x + 3.5x = 27,500, which simplifies to 27.5x = 27,500. Thus, x = 1,000. Therefore, an appropriate label for the y-axis is “Number of installations (in thousands).”
Choices A, B, and D are incorrect and may result from errors when setting up and calculating the units for the y-axis.
1 decagram = 10 grams
1,000 milligrams = 1 gram
A hospital stores one type of medicine in 2-decagram containers. Based on the information given in the box above, how many 1-milligram doses are there in one 2-decagram container?
-
Solution
Since there are 10 grams in 1 decagram, there are 2 × 10 = 20 grams in 2 decagrams. Since there are 1,000 milligrams in 1 gram, there are 20 × 1,000 = 20,000 milligrams in 20 grams. Therefore, 20,000 1-milligram doses of the medicine can be stored in a 2-decagram container.
Choice A is incorrect; 0.002 is the number of grams in 2 milligrams. Choice B is incorrect; it could result from multiplying by 1,000 and dividing by 10 instead of multiplying by both 1,000 and 10 when converting from decagrams to milligrams. Choice C is incorrect; 2,000 is the number of milligrams in 2 grams, not the number of milligrams in 2 decagrams.