John Croxley, the mayor of Black Rock, NY, is counting the number of restaurants that have opened in his town per month for the last seven months. He compiles the seven numbers into Set F, which contains the elements 4, 5, 11, 13, 16, 18, and x. If both the median and average (arithmetic mean) of Set F equal 11, what must be the value of x, the unknown number of restaurants that opened in Mayor Croxley’s town last month?
-
Solution
The formula for determining an average can be expressed by Average \(= \frac{Total}{\# \; of \; things}\).
Plug the values provided into the equation as follows: \(11 = \frac{4 + 5 + 11 + 13 + 16 + 18 + x}{7}, \; or \; 11 = \frac{67 + x}{7}\).
Multiply both sides by 7 to get 77 = 67 + x.
Subtract 67 from both sides to get x = 10, which is (B).
The chart below shows data about the number of employees at Cuda Cola, a popular beverage company.
| 2012 | 2013 | 2014 | |
| Total Employees | 1,670 | 1,890 | 2,110 |
| Percent Male | 65% | 60% | 55% |
| Percent Female | 35% | 40% | 45% |
Assuming the employee total grows at the same rate each year, and male and female percentages continue to decrease and increase by 5%, respectively, approximately how many male employees will work at Cuda Cola in 2015 ?
-
Solution
The question states that the number of employees increases at the same rate per year, so you can determine this numerical increase by subtracting one year's total from the next year's total.
Subtracting the 2013 total from the 2014 total gives 2,110 - 1,890 = 220.
To find the total in 2015, add 220 to the 2014 total: 2,110 + 220 = 2,330.
The question also states that the male percentages continue to decrease at the same rate, which, based on the data in the table, is 5% per year.
The percent male in 2014 was 55%, so the percent male in 2015 will be 50%.
50% of 2,330 can be expressed as or \(\frac{50}{100}\left(2330 \right) = 1,165\), which is (D).
McCoy Max Speed, Inc. makes custom skateboards for its customers. Two wooden skateboards and three composite skateboards cost $650. Three wooden skateboards and one composite skateboard cost $450. How much would McCoy Max Speed charge a customer who purchases five wooden skateboards and four composite skateboards?
-
Solution
If you represent the wooden skateboards with a w and the composite skateboards with a c, you can write two equations based on the information given in the question: 2w + 3c = 650 and 3w + c = 450.
It is possible to isolate one of the variables and solve these two equations by substitution, but in this case it is easier simply to stack the equations on top of each other and add them together as follows:
\(\frac{\begin{matrix} & 2w & + & 3c & = & 650 \\ + & 3w & + & c & = & 450 \end{matrix}}{\begin{matrix} & 5w & + & 4c & = & 1,100 \end{matrix}}\)
Since the question asks for the price of five wooden skateboards and four composite skateboards, the answer is (D).
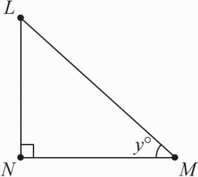
In the figure above, if y = 40 and \(\overline{LN}\) = 8, which of the following most closely approximates the length of \(\overline{MN}\) ?
-
Solution
Triangle legs LN and MN are opposite and adjacent, respectively, to ∠y.
Therefore, from SOHCAHTOA, we need to use the tangent trigonometric function.
Plugging in the values that the question gives us into the equation for tangent, we get tan 40° = \(\frac{8}{MN}\).
Now, use your calculator to determine that the length of MN most closely approximates 9.53, which is (B).
As part of a project for his cartography elective, Adam climbs several hills to create a relief map for the woods surrounding his house. He records the vertical heights of the five hills he climbed at 55 feet, 42 feet, 38 feet, 50 feet, and 48 feet. For his project, Adam must convert his measurements to inches. If 1 foot = 12 inches, what is the measurement, in inches, of the tallest hill Adam will have on his map?
-
Solution
The tallest hill that Adam measures is 55 feet high.
Since 1 foot is equivalent to 12 inches, simply multiply 55 × 12 = 660 inches, (A).
What is the value of (2x2 + 4x + 8) – (2x2 – 4x + 7) ?
-
Solution
To solve this question, rearrange the expressions (2x2 + 4x + 8) and (2x2 - 4x + 7) in order to place the similar terms next to each other.
Doing so will give you 2x2 - 2x2 + 4x - (-4x) + 8 - 7 (remember to distribute the negative sign for each of the terms in the second expression).
Simplifying this new expression will yield 0 + 8x + 1, or 8x + 1, (C).
Another way to approach this question would be to plug in a simple number for x, such as x = 2, and match your target value with the values in the answer choices.
In the inequality 37 ≤ – 2x + 1, what is the appropriate order of steps needed to solve the inequality for x ?
-
Solution
The goal here is to isolate x. Since the right-hand side of the equation is -2x + 1, you will want to subtract 1 from both sides, so eliminate (A) and (C).
To get x by itself, you will want to divide by -2, not 2, so eliminate (D) and choose (B).
Remember that when you multiply or divide across an inequality sign using a negative number, you need to flip the inequality sign in the opposite direction, as reflected in (B).
Officer Blake drives his squad car 1 mile per minute while patrolling local highways during his shift. If he has driven 480 miles by the end of his shift, how many total hours did he drive his car at the above rate?
-
Solution
Since Officer Blake drives 480 miles at a rate of 1 mile per minute, his total drive time was 480 minutes.
The question asks for Officer Blake's driving time in hours, so you need to convert those minutes into hours.
Since there are 60 minutes in 1 hour, you can divide 480 minutes by 60 to determine that Officer Blake drove for 8 hours, which is (A).
Which of the following is equivalent to the expression x4 – x3 – x2 ?
-
Solution
To solve this question, simply factor out the largest value that fits within each of the terms in the expression provided.
In this case, x4, x3, and x2 are all divisible by x2, so that is what you will want to factor out.
Doing so will leave you with x2 (x2 - x - 1), which is (D).
Dr. Goldberg, a noted dietician, mixes different solutions as part of her research into sugar substitutes. By weight, she mixes 40% of a sample of substitute A and 70% of a sample of substitute B to create substitute C. If Dr. Goldberg initially had 60 grams of substitute A and 110 grams of substitute B, then what would be the weight, in grams, of substitute C ?
-
Solution
Dr. Goldberg takes 40% of substitute A, which consists of 60 grams.
Mathematically, this can be expressed as \(\frac{40}{100}\)(60) or (0.4)(60) = 24 grams.
She takes 70% of substitute B, which consists of 110 grams.
Mathematically, this can be expressed as \(\frac{70}{100}\)(110) or (0.7)(110) = 77 grams.
Substitute C will therefore consist of 24 grams + 77 grams = 101 grams, which is (C).