Intersecting lines r, s, and t are shown below
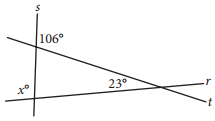
What is the value of x ?
-
Solution
The correct answer is 97. The intersecting lines form a triangle, and the angle with measure of x° is an exterior angle of this triangle. The measure of an exterior angle of a triangle is equal to the sum of the measures of the two nonadjacent interior angles of the triangle. One of these angles has measure of 23° and the other, which is supplementary to the angle with measure 106°, has measure of 180° − 106° = 74°. Therefore, the value of x is 23 + 74 = 97.
The expression above is equivalent to \frac{a}{(x+2)^{2}}, where a is a positive constant and x ≠ −2. What is the value of a ?
-
Solution
The correct answer is 2.The given expression can be rewritten as \frac{2x+6}{(x+2)^{2}}-\frac{2x+4}{(x+2)^{2}}, which is equivalent to\frac{2x+6-2x+4}{(x+2)^{2}}, or \frac{2}{(x+2)^{2}}. This is in the form \frac{a}{(x+2)^{2}}; therefore, a = 2.
y = 2x
The system of equations above has solution (x, y). What is the value of x ?
-
Solution
The correct answer is \frac{21}{4}, or 5.25. Use substitution to create a one-variable equation that can be solved for x. The second equation gives that y = 2x. Substituting 2x for y in the first equation gives 1⁄2(2x + 2x)=\frac{21}{2}. Dividing both sides of this equation by 1⁄2 yields (2x + 2x) = 21. Combining like terms results in 4x = 21. Finally, dividing both sides by 4 gives x = \frac{21}{4}= 5.25. Either 21/4 or 5.25 can be gridded as the correct answer.
2(p + 1) + 8(p – 1)1 = 5p
What value of p is the solution of the equation above?
-
Solution
The correct answer is 6⁄5, or 1.2. To solve the equation 2(p + 1) + 8(p − 1) = 5p, first distribute the terms outside the parentheses to the terms inside the parentheses: 2p + 2 + 8p − 8 = 5p. Next, combine like terms on the left side of the equal sign: 10p − 6 = 5p. Subtracting 10p from both sides yields −6 = −5p. Finally, dividing both sides by −5 gives p = 6⁄5 = 1.2. Either 6/5 or 1.2 can be gridded as the correct answer.
Maria plans to rent a boat. The boat rental costs $60 per hour, and she will also have to pay for a water safety course that costs $10. Maria wants to spend no more than $280 for the rental and the course. If the boat rental is available only for a whole number of hours, what is the maximum number of hours for which Maria can rent the boat?
-
Solution
The correct answer is 4. The equation 60h + 10 ≤ 280, where h is the number of hours the boat has been rented, can be written to represent the situation. Subtracting 10 from both sides and then dividing by 60 yields h ≤ 4.5. Since the boat can be rented only for whole numbers of hours, the maximum number of hours for which Maria can rent the boat is 4.
Alan drives an average of 100 miles each week. His car can travel an average of 25 miles per gallon of gasoline. Alan would like to reduce his weekly expenditure on gasoline by $5. Assuming gasoline costs $4 per gallon, which equation can Alan use to determine how many fewer average miles, m, he should drive each week?
-
Solution
Since gasoline costs $4 per gallon, and since Alan’s car travels an average of 25 miles per gallon, the expression \frac{4}{25} gives the cost, in dollars per mile, to drive the car. Multiplying \frac{4}{25} by m gives the cost for Alan to drive m miles in his car. Alan wants to reduce his weekly spending by $5, so setting \frac{4}{25} m equal to 5 gives the number of miles, m, by which he must reduce his driving.
Choices A, B, and C are incorrect. Choices A and B transpose the numerator and the denominator in the fraction. The fraction \frac{25}{4} would result in the unit miles per dollar, but the question requires a unit of dollars per mile. Choices A and C set the expression equal to 95 instead of 5, a mistake that may result from a misconception that Alan wants to reduce his driving by 5 miles each week; instead, the question says he wants to reduce his weekly expenditure by $5.
f(x) = 2x + 1
The function f is defined by the equation above. Which of the following is the graph of y = −f(x) in the xy-plane?
-
Solution
The graph of y = −f(x) is the graph of the equation y = −(2x + 1), or y = −2x− 1. This should be the graph of a decreasing exponential function. The y-intercept of the graph can be found by substituting the value x = 0 into the equation, as follows: y = −20 − 1 = −1 − 1 = −2. Therefore, the graph should pass through the point (0, −2). Choice C is the only function that passes through this point.
Choices A and B are incorrect because the graphed functions are increasing instead of decreasing. Choice D is incorrect because the function passes through the point (0, −1) instead of (0, −2).
At a restaurant, n cups of tea are made by adding t tea bags to hot water. If t n = +2, how many additional tea bags are needed to make each additional cup of tea?
-
Solution
When n is increased by 1, t increases by the coefficient of n, which is 1.
Choices A, C, and D are incorrect and likely result from a conceptual error when interpreting the equation.
Which of the following is equivalent to 93⁄4 ?
-
Solution
Since 9 can be rewritten as 32 , 93⁄4 properties of exponents, this can be written as 3 sup>3⁄2, which can further be rewritten as 33⁄2 (31⁄2), an expression that is equivalent to 3√3.
Choices A is incorrect; it is equivalent to 91⁄3. Choice B is incorrect; it is equivalent to 91⁄4. Choice C is incorrect; it is equivalent to 31⁄2.
The volume of right circular cylinder A is 22 cubic centimeters. What is the volume, in cubic centimeters, of a right circular cylinder with twice the radius and half the height of cylinder A?
-
Solution
The volume of right circular cylinder A is given by the expression πr2h, where r is the radius of its circular base and h is its height. The volume of a cylinder with twice the radius and half the height of cylinder A is given by π(2r)2(1⁄2)h, which is equivalent to 4πr1⁄2(1⁄2)h = 2πr2h.Therefore, the volume is twice the volume of cylinder A, or 2 × 22 = 44.
Choice A is incorrect and likely results from not multiplying the radius of cylinder A by 2. Choice B is incorrect and likely results from not squaring the 2 in 2r when applying the volume formula. Choice D is incorrect and likely results from a conceptual error.