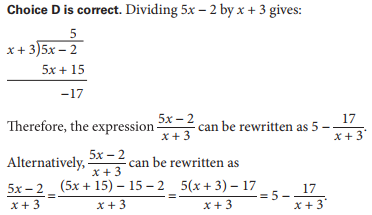ax + by = 12
2x + 8y = 60
In the system of equations above, a and b are constants. If the system has infinitely many solutions, what is the value of a⁄b?
-
Solution
The correct answer is 1⁄4 or .25. In order for a system of two linear equations to have infinitely many solutions, the two equations must be equivalent. Thus, the equation ax + by = 12 must be equivalent to the equation 2x + 8y = 60. Multiplying each side of ax + by = 12 by 5 gives 5ax + 5by = 60, which must be equivalent to 2x + 8y = 60. Since the right-hand sides of 5ax + 5by = 60 and 2x + 8y = 60 are the same, equating coefficients gives 5a = 2, or a = 2⁄5, and 5b = 8, or b = 8⁄5. Therefore, the value of a⁄b = (2⁄5) ÷ (8⁄5),which is equal to 1⁄4. Either the fraction 1⁄4 or its equivalent decimal, .25, may be gridded as the correct answer.
Alternatively, since ax + by = 12 is equivalent to 2x + 8y = 60, the equation ax + by =12 is equal to 2x + 8y = 60 multiplied on each side by the same constant. Since multiplying 2x + 8y = 60 by a constant does not change the ratio of the coefficient of x to the coefficient of y, it follows that a⁄b = 2⁄8 = 1⁄4

In the xy-plane above, O is the center of the circle, and the measure of ∠AOB is π⁄a radians. What is the value of a ?
-
Solution
The correct answer is 6. By the distance formula, the length of radius OA is \(\sqrt{(\sqrt{3})^{2}+1^{2}}=\sqrt{3+1}=2\). Thus, sin(∠AOB) = 1⁄2. Therefore, the measure of ∠AOB is 30°, which is equal to 30(\(\frac{\pi }{180}\)) = π⁄6 radians. Hence, the value of a is 6.

In the figure above, \(\overline{AE}\) || \(\overline{CD}\) and segment AD intersects segment CE at B. What is the length of segment CE ?
-
Solution
The correct answer is 12. Angles ABE and DBC are vertical angles and thus have the same measure. Since segment AE is parallel to segment CD, angles A and D are of the same measure by the alternate interior angle theorem. Thus, by the angle-angle theorem, triangle ABE is similar to triangle DBC, with vertices A, B, and E corresponding to vertices D, B, and C, respectively.Thus,\(\frac{AB}{DB}=\frac{EB}{CB}\) or \(\frac{10}{5}=\frac{8}{CB}\). It follows that CB = 4, and so CE = CB + BE = 4 + 8 =12.
2x(3x + 5) + 3(3x + 5) = ax2 + bx + c
In the equation above, a, b, and c are constants. If the equation is true for all values of x, what is the value of b ?
-
Solution
The correct answer is 19. Since 2x(3x + 5) + 3(3x + 5) = ax2 + bx + c for all values of x, the two sides of the equation are equal, and the value of b can be determined by simplifying the left-hand side of the equation and writing it in the same form as the right-hand side. Using the distributive property, the equation becomes (6x2 + 10x) + (9x + 15) = ax2 + bx + c. Combining like terms gives 6x2 + 19x + 15 = ax2 + bx + c. The value of b is the coefficient of x, which is 19.
The sales manager of a company awarded a total of $3000 in bonuses to the most productive salespeople. The bonuses were awarded in amounts of $250 or $750. If at least one $250 bonus and at least one $750 bonus were awarded, what is one possible number of $250 bonuses awarded?
-
Solution
The correct answer is 3, 6, or 9. Let x be the number of $250 bonuses awarded, and let y be the number of $750 bonuses awarded. Since $3000 in bonuses were awarded, and this included at least one $250 bonus and one $750 bonus, it follows that 250x + 750y = 3000, where x and y are positive integers. Dividing each side of 250x + 750y = 3000 by 250 gives x + 3y = 12, where x and y are positive integers. Since 3y and 12 are each divisible by 3, it follows that x = 12 − 3y must also be divisible by 3. If x = 3, then y = 3; if x = 6, then y = 2; and if x = 9, then y = 1. If x = 12, then y = 0, but this is not possible since there was at least one $750 bonus awarded. Therefore, the possible numbers of $250 bonuses awarded are 3, 6, and 9. Any of the numbers 3, 6, or 9 may be gridded as the correct answer.
The expression \(\frac{5 −2x}{x+3}\) is equivalent to which of the following?
-
Solution

Choices A and B are incorrect and may result from incorrectly canceling out the x in the expression \(\frac{5x-2}{x+3}\). Choice C is incorrect and may result from finding an incorrect remainder when performing long division.
A radioactive substance decays at an annual rate of 13 percent. If the initial amount of the substance is 325 grams, which of the following functions f models the remaining amount of the substance, in grams, t years later?
-
Solution

What is the sum of all values of m that satisfy 2m2 − 16m + 8 = 0?
-
Solution
The problem asks for the sum of the roots of the quadratic equation 2m2 − 16m + 8 = 0. Dividing each side of the equation by 2 gives m2 − 8m + 4 = 0. If the roots of m2 − 8m + 4 = 0 are s1 and s2, then the equation can be factored as m2 − 8m + 4 = (m − s1)(m − s2) = 0. Looking at the coecient of x on each side of m2 − 8m + 4 = (m − s1)(m − s2) gives −8 = −s1 − s2, or s1 + s2 = 8.
Alternatively, one can apply the quadratic formula to either 2m2 − 16m + 8 = 0 or m2 − 8m √3 and 4 + 2 √3 whose sum is 8.
Choices A, B, and C are incorrect and may result from calculation errors when applying the quadratic formula or a sign error when determining
A website uses the formula above to calculate a seller’s rating, R, based on the number of favorable reviews, F, and unfavorable reviews, N. Which of the following expresses the number of favorable reviews in terms of the other variables?
-
Solution
Multiplying each side of \(R=\frac{F}{N+F}\) by N + F gives R(N + F) = F, which can be rewritten as RN + RF = F. Subtracting RF from each side of RN + RF = F gives RN = F − RF, which can be factored as RN = F(1 − R). Finally, dividing each side of RN = F(1 − R) by 1 − R, expresses F in terms of the other variables: \(F=\frac{RN}{1-R}\).
Choices A, C, and D are incorrect and may result from calculation errors when rewriting the given equation.
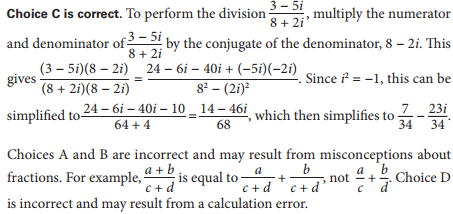
Which of the following complex numbers is equivalent to \(\frac{3−5i}{8+2i}\)? (Note: i = √−1 )
-
Solution