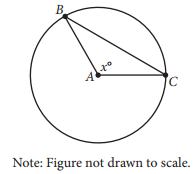
In the circle above, point A is the center and the length of arc \(\overset{\frown}{BC}\) is 2⁄5 of the circumference of the circle. What is the value of x ?
-
Solution
The correct answer is 144. In a circle, the ratio of the length of a given arc to the circle’s circumference is equal to the ratio of the measure of the arc, in degrees, to 360°. The ratio between the arc length and the circle’s circumference is given as 2⁄5. It follows that 2⁄5 = \(\frac{x}{360}\). Solving this proportion for x gives x = 144.
A start-up company opened with 8 employees. The company’s growth plan assumes that 2 new employees will be hired each quarter (every 3 months) for the first 5 years. If an equation is written in the form y ax b = + to represent the number of employees, y, employed by the company x quarters after the company opened, what is the value of b ?
-
Solution
The correct answer is 8. The number of employees, y, expected to be employed by the company x quarters after the company opened can be modeled by the equation y = ax + b, where a represents the constant rate of change in the number of employees each quarter and b represents the number of employees with which the company opened. The company’s growth plan assumes that 2 employees will be hired each quarter, so a = 2. The number of employees the company opened with was 8, so b = 8.
− x + y = −3.5
x + 3y = 9.5
If (x,y) satisfies the system of equations above, what is the value of y ?
-
Solution
The correct answer is 3⁄2. One method for solving the system of equations for y is to add corresponding sides of the two equations.Adding the left-hand sides gives (−x + y) + (x + 3y), or 4y. Adding the right-hand sides yields −3.5 + 9.5 = 6. It follows that 4y = 6. Finally, dividing both sides of 4y = 6 by 4 yields y = 6⁄4 or 3⁄2.Any of 3/2, 6/4, 9/6, 12/8 or the decimal equivalent 1.5 will be scored as correct.
The sum of −2x2 + x + 31 and 3x2 + 7x − 8 can be written in the form ax2 + bx + c, where a, b, and c are constants. What is the value of a + b + c ?
-
Solution
The correct answer is 32. The sum of the given expressions is (−2x2 + x + 31) + (3x2 + 7x − 8). Combining like terms yields x2 + 8x + 23. Based on the form of the given equation, a = 1, b = 8, and c = 23.Therefore, a + b + c = 32.
Alternate approach: Because a + b + c is the value of ax2 + bx + c when x = 1, it is possible to first make that substitution into each polynomial before adding them. When x = 1, the first polynomial is equal to −2 + 1 + 31 = 30 and the second polynomial is equal to 3 + 7 − 8 = 2.
The sum of 30 and 2 is 32.
x2 + x − 12 = 0
If a is a solution of the equation above and a > 0, what is the value of a ?
-
Solution
The correct answer is 3. The solution to the given equation can be found by factoring the quadratic expression. The factors can be determined by finding two numbers with a sum of 1 and a product of −12. The two numbers that meet these constraints are 4 and –3. Therefore, the given equation can be rewritten as (x + 4)(x − 3) = 0. It follows that the solutions to the equation are x = −4 or x = 3. Since it is given that a > 0, a must equal 3.
g(x) = 2x −1
h(x) = 1 – g(x)
The functions g and h are defined above. What is the value of h(0)?
-
Solution
Since h(x) = 1 − g(x), substituting 0 for x yields h(0) = 1 − g(0). Evaluating g(0) gives g(0) = 2(0) − 1 = −1. Therefore, h(0) = 1 − (−1) = 2.
Choice A is incorrect. This choice may result from an arithmetic error. Choice B is incorrect. This choice may result from incorrectly evaluating g(0) to be 1. Choice C is incorrect. This choice may result from evaluating 1 − 0 instead of 1 − g(0).
y = x2 +3 −7
y = −5 + 8 = 0
How many solutions are there to the system of equations above?
-
Solution
The second equation of the system can be rewritten as y = 5x − 8. Substituting 5x − 8 for y in the first equation gives 5x − 8 = x2 + 3x − 7. This equation can be solved as shown below:
x2 + 3x − 7 − 5x + 8 = 0
x2 − 2x + 1 = 0
(x − 1)2 = 0
x = 1
Substituting 1 for x in the equation y = 5x − 8 gives y = −3. Therefore, (1, −3) is the only solution to the system of equations.
Choice A is incorrect. In the xy-plane, a parabola and a line can intersect at no more than two points. Since the graph of the first equation is a parabola and the graph of the second equation is a line, the system cannot have more than 2 solutions. Choice B is incorrect. There is a single ordered pair (x, y) that satisfies both equations of the system. Choice D is incorrect because the ordered pair (1, −3) satisfies both equations of the system.
Oil and gas production in a certain area dropped from 4 million barrels in 2000 to 1.9 million barrels in 2013. Assuming that the oil and gas production decreased at a constant rate, which of the following linear functions f best models the production, in millions of barrels, t years after the year 2000?
-
Solution
It is assumed that the oil and gas production decreased at a constant rate.Therefore, the function f that best models the production t years after the year 2000 can be written as a linear function, f(t) = mt + b, where m is the rate of change of the oil and gas production and b is the oil and gas production, in millions of barrels, in the year 2000. Since there were 4 million barrels of oil and gas produced in 2000, b = 4. The rate of change, m, can be calculated as \(\frac{4 − 1.9}{0 − 13}=-\frac{2.1}{13}\), which is equivalent to − \(\frac{21}{130}\), the rate of change in choice C.
Choices A and B are incorrect because each of these functions has a positive rate of change. Since the oil and gas production decreased over time, the rate of change must be negative. Choice D is incorrect. This model may result from misinterpreting 1.9 million barrels as the amount by which the production decreased.
If \(\frac{2a}{b}\) = 1⁄2, what is the value of b⁄a?
-
Solution
Dividing both sides of equation \(\frac{2a}{b}\) = 1⁄2 by 2 gives a⁄b = 1⁄4. Taking the reciprocal of both sides yields b⁄a = 4.
Choice A is incorrect. This is the value of \(\frac{a}{2b}\), not b⁄a . Choice B is incorrect. This is the value of a⁄b, not b⁄a. Choice C is incorrect. This is the value of \(\frac{b}{2a}\), not b⁄a.
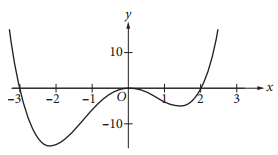
Which of the following could be the equation of the graph above?
-
Solution
The x-coordinates of the x-intercepts of the graph are –3, 0, and 2. This means that if y = f (x) is the equation of the graph, where f is a polynomial function, then (x + 3), x, and (x − 2) are factors of f. Of the choices given, A and B have the correct factors. However, in choice A, x is raised to the first power, and in choice B, x is raised to the second power. At x = 0, the graph touches the x-axis but doesn’t cross it. This means that x, as a factor of f, is raised to an even power. If x were raised to an odd power, then the graph would cross the x-axis. Alternatively, in choice A, f is a third-degree polynomial, and in choice B, f is a fourth-degree polynomial. The y-coordinates of points on the graph become large and positive as x becomes large and negative; this is consistent with a fourth-degree polynomial, but not with a third-degree polynomial. Therefore, of the choices given, only choice B could be the equation of the graph.
Choice A is incorrect. The graph of the equation in this answer choice has the correct factors. However, at x = 0 the graph of the equation in this choice crosses the x-axis; the graph shown touches the x-axis but doesn’t cross it. Choices C and D are incorrect and are likely the result of misinterpreting the relationship between the x-intercepts of a graph of a polynomial function and the factors of the polynomial expression.