Jaime is preparing for a bicycle race. His goal is to bicycle an average of at least 280 miles per week for 4 weeks. He bicycled 240 miles the first week, 310 miles the second week, and 320 miles the third week. Which inequality can be used to represent the number of miles, x, Jaime could bicycle on the 4th week to meet his goal?
-
Solution
Jaime’s goal is to average at least 280 miles per week for 4 weeks. If T is the total number of miles Jamie will bicycle for 4 weeks, then his goal can be represented symbolically by the inequality:T⁄4, or equivalently T ≥ 4(280). The total number of miles Jamie will bicycle during this time is the sum of the distances he has completed and has yet to complete. Thus T = 240 + 310 + 320 + x. Substituting this expression into the inequality T ≥ 4(280) gives 240 + 310 + 320 + x ≥ 4(280). Therefore, choice D is the correct answer.
Choices A, B, and C are incorrect because they do not correctly capture the relationships between the total number of miles Jaime will ride his bicycle (240 + 310 + 320 + x) and the minimum number of miles he is attempting to bicycle for the four weeks (280 + 280 + 280 + 280).
If √x + √9 = \(\sqrt{64}\) , what is the value of x ?
-
Solution
The two numerical expressions in the given equation can be simplified as √9 = 3 and \(\sqrt{64}\) = 8, so the equation can be rewritten as √x + 3 = 8, or √x = 5. Squaring both sides of the equation gives x = 25.
Choice A is incorrect and may result from a misconception about how to square both sides of √x = 5 to determine the value of x. Choice B is incorrect. The value of √x, not x, is 5. Choice D is incorrect and represents a misconception about the properties of radicals. While it is true that 55 + 9 = 64, it is not true that \(\sqrt{55}\) + √9 = 64.
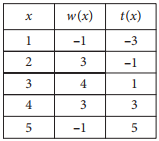
The table above shows some values of the functions w and t. For which value of x is w(x) + t(x) = x ?
-
Solution
This question can be answered by making a connection between the table and the algebraic equation. Each row of the table gives a value of x and its corresponding values in both w(x) and t(x). For instance, the first row gives x = 1 and the corresponding values w(1) = −1 and t(1) = −3. The row in the table where x = 2 is the only row that has the property x = w(x) + t(x): 2 = 3 + (−1). Therefore, choice B is the correct answer.
Choice A is incorrect because when x = 1, the equation w(x) + t(x) = x is not true. According to the table, w(1) = −1 and t(1) = −3. Substituting the values of each term when x = 1 gives −1 + (−3) = 1, an equation that is not true. Choice C is incorrect because when x = 3, the equation w(x) + t(x) = x is not true. According to the table, w(3) = 4 and t(3) = 1. Substituting the values of each term when x = 3 gives 4 + 1 = 3, an equation that is not true. Choice D is incorrect because when x = 4, the equation w(x) + t(x) = x is not true. According to the table, w(4) = 3 and t(4) = 3. Substituting the values of each term when x = 4 gives 3 + 3 = 4, an equation that is not true.
A bricklayer uses the formula n = 7 \(\iota\) h to estimate the number of bricks, n, needed to build a wall that is \(\iota\) feet long and h feet high. Which of the following correctly expresses \(\iota\) in terms of n and h ?
-
Solution
By properties of multiplication, the formula n = h \(\iota\) 7 can be rewritten as n = (7h)\(\iota\). To solve for in terms of n and h, divide both sides of the equation by the factor 7h.
Solving this equation for \(\iota\) gives \(\iota=\frac{n}{7h}\).
Choices A, B, and D are incorrect and may result from algebraic errors when rewriting the given equation.
If x = 2⁄3 y = and y = 18, what is the value of 2x − 3?
-
Solution
Substituting the given value of y = 18 into the equation x = 2⁄3 yields ? = (2⁄3) (18), or x = 12. The value of the expression 2x − 3 when x = 12 is 2(12) − 3 = 21.
Choice B is incorrect. If 2x − 3 = 15, then adding 3 to both sides of the equation and then dividing both sides of the equation by 2 yields x = 9. Substituting 9 for x and 18 for y into the equation x = 2⁄3 y yields 9 = 2⁄318=12, which is false. Therefore, the value of 2x − 3 cannot be 15. Choices C and D are also incorrect. As with choice B, assuming the value of 2x – 3 is 12 or 10 will lead to a false statement.
Which of the following is the graph of the equation y =2 −5 x in the xy-plane?
-
Solution
Choice D is correct. In the xy-plane, the graph of the equation y = mx + b, where m and b are constants, is a line with slope m and y-intercept (0, b). Therefore, the graph of y = 2x − 5 in the xy-plane is a line with slope 2 and a y-intercept (0, −5). Having a slope of 2 means that for each increase in x by 1, the value of y increases by 2. Only the graph in choice D has a slope of 2 and crosses the y-axis at (0, −5). Therefore, the graph shown in choice D must be the correct answer.
Choices A, B, and C are incorrect. The graph of y = 2x − 5 in the xy-plane is a line with slope 2 and a y-intercept at (0, −5). The graph in choice A crosses the y-axis at the point (0, 2.5), not (0,−5), and it has a slope of 1⁄2, not 2. The graph in choice B crosses the y-axis at (0, −5); however,the slope of this line is −2, not 2. The graph in choice C has a slope of 2; however, the graph crosses the y-axis at (0, 5), not (0, −5).
4x2 − 9 = (px + t)(px – t)
In the equation above, p and t are constants. Which of the following could be the value of p ?
-
Solution
Choice A is correct. The right side of the equation can be multiplied using the distributive property: (px + t)(px − t) = p2 x2− ptx + ptx − t2. Combining like terms gives p2x2− t2. Substituting this expression for the right side of the equation gives 4x2 − 9 = p2 x2 − t2, where p and t are constants. This equation is true for all values of x only when 4 = p2 and 9 = t2. If 4 = p2, then p = 2 or p = −2. Therefore, of the given answer choices, only 2 could be the value of p.
Choices B, C, and D are incorrect. For the equation to be true for all values of x, the coefficients of x2 on both sides of the equation must be equal; that is, 4 = p2. Therefore, the value of p cannot be 3, 4, or 9.
What is the sum of the complex numbers 2 + 3i and 4 + 8i, where i = \(\sqrt{-1}\)?
-
Solution
For a complex number written in the form a + bi, a is called the real part of the complex number and b is called the imaginary part. The sum of two complex numbers, a + bi and c + di, is found by adding real parts and imaginary parts, respectively; that is, (a + bi) + (c + di) = (a + c) + (b + d)i. Therefore, the sum of 2 + 3i and 4 + 8i is (2 + 4) + (3 + 8)i = 6 + 11i.
Choice A is incorrect and is the result of disregarding i and adding all parts of the two complex numbers together, 2 + 3 + 4 + 8 = 17. Choice B is incorrect and is the result of adding all parts of the two complex numbers together and multiplying the sum by i. Choice D is incorrect and is the result of multiplying the real parts and imaginary parts of the two complex numbers, (2)(4) = 8 and (3)(8) = 24, instead of adding those parts together.
A gardener buys two kinds of fertilizer. Fertilizer A contains 60% filler materials by weight and Fertilizer B contains 40% filler materials by weight. Together, the fertilizers bought by the gardener contain a total of 240 pounds of filler materials. Which equation models this relationship, where x is the number of pounds of Fertilizer A and y is the number of pounds of Fertilizer B?
-
Solution
Since Fertilizer A contains 60% filler materials by weight, it follows that x pounds of Fertilizer A consists of 0.6x pounds of filler materials. Similarly, y pounds of Fertilizer B consists of 0.4y pounds of filler materials. When x pounds of Fertilizer A and y pounds of Fertilizer B are combined, the result is 240 pounds of filler materials. Therefore, the total amount, in pounds, of filler materials in a mixture of x pounds of Fertilizer A and y pounds of Fertilizer B can be expressed as 0.6x + 0.4y = 240.
Choice A is incorrect. This choice transposes the percentages of filler zaterials for Fertilizer A and Fertilizer B. Fertilizer A consists of 0.6x pounds of filler materials and Fertilizer B consists of 0.4y pounds of filler materials. Therefore, 0.6x + 0.4y is equal to 240, not 0.4x + 0.6y. Choice C is incorrect. This choice incorrectly represents how to take the percentage of a value mathematically. Fertilizer A consists of 0.6x pounds of filler materials, not 60x pounds of filler materials, and Fertilizer B consists of 0.4y pounds of filler materials, not 40y pounds of filler materials. Choice D is incorrect. This choice transposes the percentages of filler materials for Fertilizer A and Fertilizer B and incorrectly represents how to take the percentage of a value mathematically.
Salim wants to purchase tickets from a vendor to watch a tennis match. The vendor charges a one-time service fee for processing the purchase of the tickets. The equation T = 15n + 12 represents the total amount T, in dollars, Salim will pay for n tickets. What does 12 represent in the equation?
-
Solution
The total amount T, in dollars, Salim will pay for n tickets is given by T = 15n + 12, which consists of both a per-ticket charge and a one-time service fee. Since n represents the number of tickets that Salim purchases, it follows that 15n represents the price, in dollars, of n tickets. Therefore, 15 must represent the per-ticket charge. At the same time, no matter how many tickets Salim purchases, he will be charged the $12 fee only once. Therefore, 12 must represent the amount of the service fee, in dollars.
Choice A is incorrect. Since n represents the total number of tickets that Salim purchases, it follows that 15n represents the price, in dollars, of n tickets, excluding the service fee.Therefore, 15, not 12, must represent the price of 1 ticket. Choice C is incorrect. If Salim purchases only 1 ticket, the total amount, in dollars, Salim will pay can be found by substituting n = 1 into the equation for T. If n = 1, T = 15(1) + 12 = 27. Therefore, the total amount Salim will pay for one ticket is $27, not $12. Choice D is incorrect. The total amount, in dollars, Salim will pay for n tickets is given by 15n + 12. The value 12 represents only a portion of this total amount. Therefore, the value 12 does not represent the total amount, in dollars, for any number of tickets.