Which of the following expressions is equivalent to \(\left(4s \right)^{\frac{1}{3}}\)?
-
Solution
Taking a number to the 1⁄3 power is the same as taking the cube root of the number.
Therefore, \(\left(4s \right)^{\frac{1}{3}} = \sqrt[3]{4s}\).
The correct answer is (D).
A shortcut is to just raise the coefficient to the power, and then use POE.
Since only one answer starts with \(\sqrt[3]{4}\), that one must be correct.
Another approach is to plug in a number for s and let the calculator do the hard work, though that would not help in this section since calculator use is not permitted.
Newton’s law of gravitation describes the strength of the force F between two objects with masses M and m separated by a distance of r units and is defined as. F = \(\frac{GMm}{r^{2}}\). Which of the following gives the value of Newton’s gravitational constant G, in terms of F, M, m, and r ?
-
Solution
Plugging In could work on this one, but calculators aren't permitted.
Since the equation is fairly simple, solving may be a better approach.
Multiply both sides of the equation by r2 to get Fr2 = GMm.
Divide both sides of the equation by Mm to get \(\frac{Fr^{2}}{Mm} = G\).
The correct answer is (B).
During the month of July, the number of units, y, of a certain product sold per day can be modeled by the function y = -3.65x + 915, where x is the average daily temperature in degrees Fahrenheit. Which of the following statements must be true?
-
Solution
Whenever there are variables in the question, think Plugging In.
The answers refer to what happens when the temperature, x, increases, so plug in more than one value of x.
Plug in x = 1 into the equation to get y = -3.65(1) + 915 = -3.65 + 915 = 911.35.
Next plug in x = 2 to get y = -3.65(2) + 915 = -7.3 + 915 = 907.7.
As average daily temperature, x, increased, the number of units sold, y, decreased.
Therefore, the correct answer is (A).
When a virus breaks out, each infected person can infect multiple new people. In a particularly bad flu outbreak at an elementary school, the number of infected people triples each day in the first school week of January. If 5 people were sick with the flu on Monday, which of the following equations best predicts the number of infected people, I(d), d days after Monday?
-
Solution
Whenever there are variables in the question and in the answer choices, think Plugging In.
Let d = 2. On the first day after Monday, 5 × 3 = 15 people will be infected.
On the second day after Monday, 15 × 3 = 45 people will be infected.
Therefore, when d = 2, the result is 45.
Plug 2 in for d in the answer choices to see which answer equals the target number of 45.
Choice (A) becomes 5 × 3(22) = 5 × 12 = 60.
This does not match the target number, so eliminate (A).
Choice (B) becomes 5 × 23 = 40.
Eliminate (B).
Choice (C) becomes 5 × 32 = 45.
Keep (C), but check the remaining choice just in case.
Choice (D) becomes 5 × 9(5) = 225.
Eliminate (D), and choose (C).
David is planning a dinner for his birthday. At one restaurant, the cost per person for dinner is $15, with an additional one-time set-up charge of $35. David has a maximum budget of $150. If p represents the number of people (including David) who will attend the dinner, which of the following inequalities represents the number of people who can attend within budget?
-
Solution
Subtract the one-time set-up charge from David's budget first: 150 - 35 = 115.
Calculate the number of people David can invite as follows: 115 ÷ 15 = 7.6.
David can invite at most 7 people (including himself), so p ≤ 7.
In (A), 15p ≤ 185, so p ≤ 12.3 or p ≤ 12.3.
Eliminate (A).
Solve for p in (B) as follows: add 15p to both sides to get 15p + 35 ≤ 150, so 15p ≤ 115 and p ≤ 7.6.
The correct answer is (B).
Which of the following lines contains all points equidistant from the points (0, 4) and (8, 0) in the xy-plane?
-
Solution
First, start with a sketch of the two points to see what the line in question might look like.

The point directly between the two points will definitely be on the line, so find the midpoint of the two points.
Midpoint = \(\left ( \frac{x_{1} + x_{2}}{2}, \frac{y_{1} + y_{2}}{2} \right ) = \left ( \frac{0 + 8}{2}, \frac{4 + 0}{2} \right ) = \left ( 4 , 2 \right )\).
Check this point in the answer choices and eliminate any that do not contain it.
Choice (A) becomes 2(2) = -4 + 8 or 4 = 4, which is true.
Choice (B) becomes 2(2) = 4, and (C) becomes 2 = 2(4) - 6 or 2 = 8 - 6.
These are also true, but (D) becomes 2 = -2(4), which is false.
Eliminate (D).
To sketch the remaining equations, rewrite them in slope-intercept form of the equation y = mx + b, where m is the slope and b is the y-intercept.
Choice (A) becomes y = -1⁄2x + 4, (B) becomes y = 1⁄2x, and (C) is already in the right form.
Now sketch the graphs of each of these on the xy-plane.

The line in (A) contains both the given points, but all the points to the left of (0, 4) are closer to that point and all those to the right of (8, 0) are closer to it.
So eliminate (A).
Many points on line (B) are also clearly closer to one or the other of the given points, so eliminate (B).
Line (C) appears to be perpendicular to the line formed by the two given points, and this is in fact what will make all the points on a line equidistant from 2 given points.
Therefore, the correct answer is (C).
If r = (1⁄2a + b)2 and s = -4ab + 3b, what is r – 2s in terms of a and b ?
-
Solution
Whenever there are variables in the question and in the answers, think Plugging In.
If a = 2 and b = 3, r = [1⁄2(2) + 3]2 = (1 + 3)2 = 16, and s = -4(2)(3) + 3(3) = -24 + 9 = -15.
The expression r - 2s becomes 16 - 2(-15) = 16 + 30 = 46.
Plug 2 in for a and 3 in for b in each of the answers to see which answer equals the target number of 46.
Choice (A) becomes 1⁄4(22) + 32 - 7(2)(3) - 6(3) = 1 + 9 - 42 - 18 = -50.
This does not match the target number, so eliminate (A).
Choice (B) becomes 1⁄4(22) + 32 - 7(2)(3) + 6(3) = 1 + 9 - 42 + 18 = -14.
Eliminate (B).
Choice (C) becomes 1⁄4(22) + 32 + 9(2)(3) - 6(3) = 1 + 9 + 54 - 18 = 46.
Keep (C), but check (D) just in case it also works.
Choice (D) is the same as (C) except for the coefficient on the a2 term, so it can't equal 46.
Eliminate (D).
The correct answer is (C).
For which of the following values of w does \(\sqrt[4]{16w^{3}x^{\frac{9}{w}}} = \left ( 2 \right )\left ( 3^{\frac{3}{4}} \right )\left ( x^{\frac{3}{4}} \right )\) ?
-
Solution
Taking the 4th root of a number is the same as taking the number to the 1⁄4 power.
Therefore, the equation can be rewritten as \(2w^{\frac{3}{4}}x^{\frac{9}{4w}} = \left ( 2 \right )\left ( 3^{\frac{3}{4}} \right )\left ( x^{\frac{3}{4}} \right )\).
Divide both sides by 2 to get \(w^{\frac{3}{4}}x^{\frac{9}{4w}} = \left ( 3^{\frac{3}{4}} \right )\left ( x^{\frac{3}{4}} \right )\).
Therefore, in the equation \(w^{\frac{3}{4}} = \left ( 3^{\frac{3}{4}} \right) \;\; and \;\; x^{\frac{9}{4w}} = \left ( x^{\frac{3}{4}} \right )\),
so w = 3. The correct answer is (B).
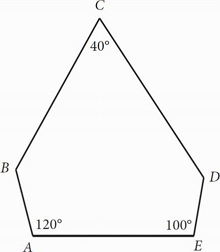
Note: Figure not drawn to scale.
In the figure above, ∠ABC ≅ ∠CDE. Which of the following is true?
-
Solution
Label the congruent angles, ∠ABC and ∠CDE, as such.
In order to find the measure of those angles, use the formula 180(n - 2), where n is the number of sides, to determine the sum of the interior angles of the figure.
Because the figure has five sides, plug 5 in for n to get 180(5 - 2), or 180(3), which equals 540. Subtract 120 to get 420.
Subtract 100 to get 320. Subtract 40 to get 280. Since the two remaining angles are congruent, divide by 2 to find that the two unlabeled angles are both equal to 140.
Because ∠ABC and ∠BCD have a combined measure of 180,\(\overline{AB} \;\; and \;\; \overline{CD}\) are parallel.
Therefore, (A) accurately describes the relationships in the figure.
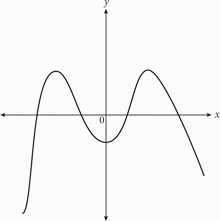
The figure above shows the graph in the xy-plane of the function g. How many distinct real roots does g have?
-
Solution
A root of the equation is the same as an x-intercept.
In the graph, the function crosses the x-axis at 4 points.
Therefore, the correct answer is (D).

